Consider a body moving in a straight line. Now, let’s analyze its motion mathematically.
For uniform velocity
If the body covers distance $s$ with uniform velocity $u$ in a time $t$, then the ratio of the displacement $s$ to the time taken $t$ i.e. $\frac{s}{t}$ is the average velocity of the body. \[u=\frac{s}{t}\]
It has both magnitude and direction, so it is a vector quantity. And, \[\text{distance covered} = \text{uniform velocity} × \text{time}\] \[s=ut\text{___(1)}\] Graphically,
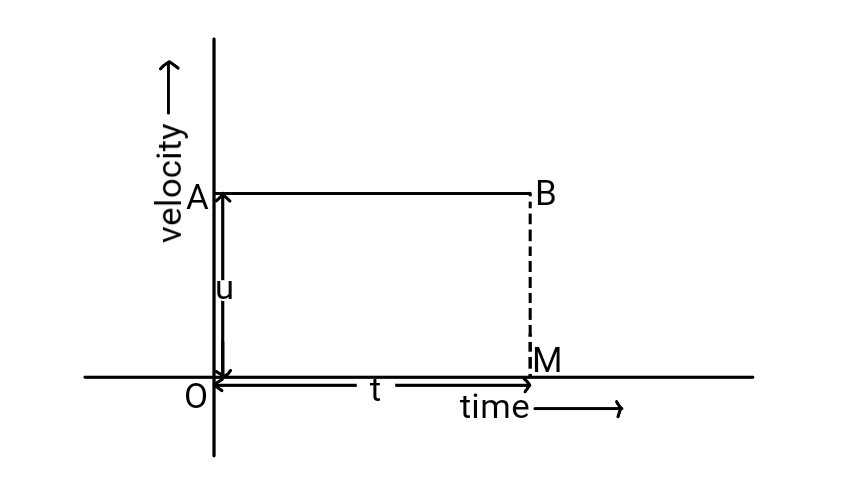
From the graph above, the distance covered is equal to the area of $OABM$. \[\text{Area of OABM}=OA×OM\] \[\therefore s=ut\]
Suppose a moving particle is at a point $A$, whose distance from the origin $O$ is $x_1$ at time $t_1$. Then, at time $t_2$, let it be at a point $B$ which is at a distance $x_2$ from $O$. Then, the displacement of the particle during the time interval $(t_2 – t_1)$ is $AB = x_2 – x_1$.

If $∆x$ denotes the change in displacement and $∆t$ denotes the change in time, then, \[∆x=x_2-x_1\] \[∆t=t_2-t_1\]
Therefore, the average velocity $u$ of the particle is \[u=\frac{∆x}{∆t}=\frac{x_2-x_1}{t_2-t_1}\]
The average velocity describes the overall effect associated with the entire distance from $A$ to $B$ and the whole time interval $t_2 – t_1$. This does not tell us about the velocity of the particle at any particular instance or position. To know this, instantaneous velocity is taken.
Mathematical Analysis of Uniform Motion
Suppose a moving particle is at a point $A$ which is at a distance $x$ from the origin $O$ at time $t$. After time $t+∆t$ (where $∆t$ is a small increment in time $t$), suppose it reaches to a point $B$ which is at a distance $x+∆x$ from $O$, where $∆x$ is the small increment in $x$ during the interval $∆t$.

We have, \[\text{Average Velocity}=\frac{∆x}{∆t}\]
If $∆t$ is made smaller and smaller, $B$ comes closer and closer to $A$. If $∆t$ approaches or tends to $0$, the ratio $\frac{∆x}{∆t}$ may or may not have a value. To have a definite value, $∆x$ should be small enough as compared to $∆t$. Therefore, $\frac{∆x}{∆t}$ may have some value as $∆t\to 0$ or as $∆x\to 0$ (or as $B\to A$). This value is the velocity of the particle at the particular instance $t$ or at the point $A$, and is called the instantaneous velocity of the particle at time $t$ or at the point $A$.
If $u$ is the instantaneous velocity of the particle at a point, then \[u=\lim_{∆t \to 0} \frac{∆x}{∆t}\]
In calculus, \[u=\frac{dx}{dt}=\lim_{∆t \to 0} \frac{∆x}{∆t}=\dot{x}\]
The non-negative numerical value or absolute value of the velocity can be defined as speed. It has only magnitude but no direction. So, it is a scalar.
If a particle covers equal distances in equal interval of times, then the particle is said to have uniform velocity or uniform speed.
Geometrically,

The average velocity may be geometrically represented by the slope of the chord $AB$. And, the instantaneous velocity at $A$ is represented by the slope of the tangent at $A$.
For Uniformly Accelerated Motion
The velocity of a moving particle may increase or decrease from point to point. If the velocity goes on increasing, then the particle is said to be accelerating. If it goes on decreasing, then the particle is said to be decelerating or retarding or have a negative acceleration.
If the rate of change of velocity is constant, then the moving particle is said to have a uniform acceleration. Let $v_1$ and $v_2$ be the velocities of a moving particle at time $t_1$ and $t_2$ respectively, then the average acceleration is \[a=\frac{v_2-v_1}{t_2-t_1}=\frac{∆v}{∆t}\]
Where, $∆v$ denotes the change in velocity and $∆t$ denotes the change in time.
If $∆t$ is very small or tends to zero and the ratio $\frac{∆v}{∆t}$ has a value, then this ratio gives the instantaneous acceleration of the moving particle at a given time. \[a=\lim_{∆t \to 0} \frac{∆v}{∆t}\]
In calculus, \[a=\frac{dv}{dt}=\frac{d}{dt}\left(\frac{dx}{dt}\right)=\frac{d^2x}{dt^2}=\ddot{x}\]
Mathematical Analysis of Uniformly Accelerated Motion
Let the body starts to move with an initial velocity $u$ and with uniform acceleration $a$ for time $t$. If $v$ is the final velocity of the body, then, \[a=\frac{v-u}{t}\] \[at=v-u\] \[∴v=u+at\text{ ___(2)}\] Graphically,
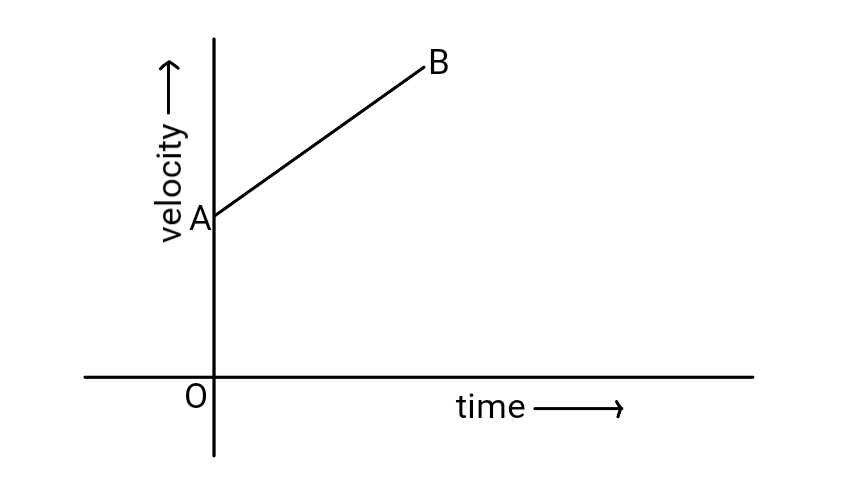
Draw $AL\perp BM$.
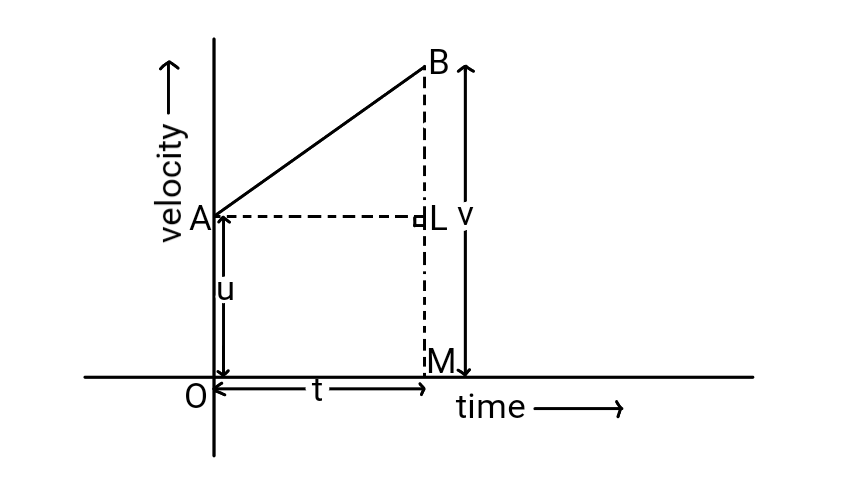
Here, the acceleration of the body is given by the slope of $AB$. \[\text{Acceleration}=\text{Slope of AB}\] \[∴a=\frac{BL}{AL}\] \[BL=BM-LM=BM-OA=v-u\] and, \[AL=OM=t\] Thus, \[a=\frac{v-u}{t}\] \[∴v=u+at\]
In calculus, \[a=\frac{dv}{dt}\] \[dv=adt\] \[\text{Integrating, } \int_u^v dv=\int_0^t a\;dt\] \[\left[v\right]_u^v=a\left[t\right]_0^t\] \[v-u=at\]\[∴v=u+at\]
If $s$ is the distance covered by the body. Then, \[\text{Distance covered}= \text{average velocity} × time\] \[s=\left(\frac{u+v}{2}\right)t\text{ ___(3)}\]
From $(2)$ and $(3)$, \[s=\left(\frac{u+u+at}{2}\right)t\] \[s=\left(\frac{2u+at}{2}\right)t\] \[∴s=ut+\frac{1}{2}at^2\text{ ___(4)}\]
Graphically
\[\text{Distance covered} = \text{Area of trapezium OABM}\] \[s=\text{Area of OALM} + \text{Area of ABL}\] \[s=OA×OM+\frac{1}{2}BL×AL\] \[s=OA×OM+\frac{1}{2}\frac{BL}{AL}(AL)^2\] \[∴s=ut+\frac{1}{2}at^2\]
In calculus, \[v=u+at\] \[\frac{ds}{dt}=u+at\] \[ds=udt+atdt\] \[\text{Integrating, } \int_0^s ds=u \int_0^t dt+ a \int_0^t tdt\] \[\left[s\right]_0^s=u\left[t\right]_0^t+a\left[\frac{t^2}{2}\right]_0^t\] \[∴s=ut+\frac{1}{2}at^2\] We have, \[v=u+at\] \[\text{Squaring both sides,}\] \[v^2=(u+at)^2\] \[v^2=u^2+2uat+u^2t^2\] \[v^2=u^2+2a\left(ut+\frac{1}{2}at^2\right)\] \[∴v^2=u^2+2as\text{ ___(5)}\]
Graphically
\[\text{Distance Covered}=\text{Area of trapezium OABM}\] \[s=\frac{1}{2}(OA+BM)×OM\] \[s=\frac{1}{2}(OA+BM)×AL\] \[s=\frac{1}{2}(OA+BM)×\frac{AL}{BL}×BL\] \[s=\frac{1}{2}(OA+BM)×\frac{AL}{BL}×(BM-LM)\] \[s=\frac{1}{2}(u+v)×\frac{1}{a}×(v-u)\] \[2as=v^2-u^2\] \[∴v^2=u^2+2as\]
In calculus, \[a=\frac{dv}{dt}=\frac{dv}{ds}×\frac{ds}{dt}=v\frac{dv}{ds}\] \[ads=vdv\] \[a\int_0^s ds=\int_u^v v\;dv\] \[a\left[s\right]_0^s=\left[\frac{v^2}{2}\right]_u^v\] \[as=\frac{v^2}{2}-\frac{u^2}{2}\] \[2as=v^2-u^2\] \[∴v^2=u^2+2as\]
Distance covered by a uniformly accelerated body in a particular second
Let a body starts to move with an initial velocity $u$ and with a uniform acceleration $a$. Then, \[\text{distance covered in }n^{th}\text{ second}\] \[S_n^{th}=S_n-S_{n-1}\text{ ___(a)}\] \[S_n \text{ is the distance covered in n seconds}\] \[S_{n-1} \text{ is the distance covered in n-1 seconds}\]
Now, \[S_n=un+\frac{1}{2}an^2\text{ ___(b)}\] and, \[S_{n-1}=u(n-1)+\frac{1}{2}a(n-1)^2\text{ ___(c)}\]
From $(a)$, $(b)$ and $(c)$, \[S_n^{th}=un+\frac{1}{2}an^2-u(n-1)-\frac{1}{2}a(n-1)^2\] \[S_n^{th}=un+\frac{1}{2}an^2-u(n-1)-\frac{1}{2}a(n^2-2n+1)\] \[S_n^{th}=un+\frac{1}{2}an^2-un+u-\frac{1}{2}an^2+an-\frac{a}{2}\] \[S_n^{th}=u+an-\frac{a}{2}\] \[∴S_n^{th}=u+\frac{a}{2}(2n-1)\]
Alternative: If $OB$ and $OA$ are the distances covered by a particle in time $n$ and $(n-1)$ sec respectively, then $AB$ is the distance covered by the body in $n^{\text{th}}$ second.
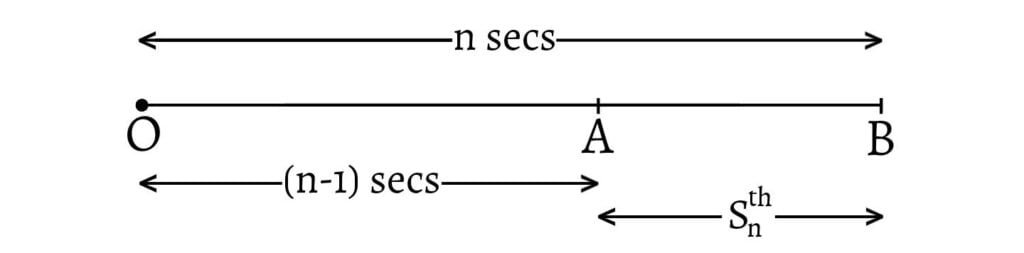
\[v=u+at=\frac{ds}{dt}\] \[∴ds=u\;dt+at\;dt\]
Integrating within limits $x = OA$ to $OB$ and $t = (n-1)$ to $n$, \[\int_{OA}^{OB} ds = u\int_{n-1}^n u\;dt + a\int_{n-1}^n t\;dt\] \[\left[s\right]_{OA}^{OB}=u\left[t\right]_{n-1}^n+a\left[\frac{t^2}{2}\right]_{n-1}^n\] \[OB-OA=u\left[n-(n-1)\right]+\frac{a}{2}\left[n^2-(n-1)^2\right]\] \[AB=u+\frac{a}{2}\left[n^2-n^2+2n-1\right]\] \[∴S_n^{th}=u+\frac{a}{2}(2n-1)\]
Therefore, the distances covered by the particle in the first, second, third, …, $n^{\text{th}}$ seconds are, \[u+\frac{a}{2} \text{, } u+\frac{3a}{2} \text{, } u+\frac{5a}{2} \text{, …, } u+\frac{a}{2}(2n-1)\]
This sequence is an arithmetic progression whose first term is $u+\frac{a}{2}$ and the common difference is $a$. Hence, if a particle moves with a uniform acceleration, the distances covered in successive seconds form an Arithmetic Progression with common difference, the acceleration.
Motion Under Gravity
Every object fall to the surface of the earth if left at certain height above the garden. A feather may fall slowly than a stone but it is only due to the presence of air resistance. Every object, whether it is small or huge, falls vertically downwards with a constant acceleration. This constant acceleration at a particular place is known as acceleration due to gravity. It is denoted by $g$. This acceleration due to gravity varies from place to place due to many factors such as height or depth from the surface of the earth, rotation of the earth, etc.
Know more : Variation of Acceleration due to Gravity
The value of $g$ near to the surface of the earth is $9.8$ $\text{ms}^{-2}$. If the air resistance is neglected and the height is kept small as compared to the radius of the earth, then the motion of the body is known as freefall. When a body falls, its acceleration is taken as positive and if it is moving upwards, its acceleration is taken to be negative.
Equations
If the body is falling down, then $a=g$. \[v=u+gt\] \[h=ut+\frac{1}{2}gt^2 \text{ [} h\text{=Height]}\] \[v^2=u^2+2gh\]
If the body is moving upwards, then $a=-g$. \[v=u-gt\] \[h=ut-\frac{1}{2}gt^2\] \[v^2=u^2-2gh\]
Motion Down An Inclined Plane
When a body is allowed to move down or up a smooth plane inclined at an angle $θ$ and free from any resistance, then the acceleration of the body depends upon the angle of inclination $θ$.

The rate at which the body moves down an inclined plane is found to be equal to the acceleration due to gravity times the sine of the inclination of the plane to the horizon i.e. $g\sin θ$. It is taken positive if the body moves down and negative if it moves up. The other component of the acceleration due to gravity $(g\cos θ)$ which is in a direction normal to the plane is neutralized by the normal reaction of the body.
Equations
For a body sliding downwards, $a=g\sin θ$. \[v=u+g\sin θt\] \[l=ut+\frac{1}{2}g\sin θt^2 \text{ [} l \text{=length]}\] \[v^2=u^2+2g\sin θl\]
For a body moving upwards, $a=-g\sin θ$. \[v=u-g\sin θt\] \[l=ut-\frac{1}{2}g\sin θ t^2\] \[v^2=u^2-2g\sin θl\]
More on Kinematics

