The most common application of derivative is that we can use it as the rate measure. Consider a continuous function $y=ƒ(x)$. Let $\Delta x$ be a small change in $x$ and $\Delta y$ be the corresponding change in $y$. Then, \[\frac{\Delta y}{\Delta x}=\frac{ƒ(x+\Delta x)-ƒ(x)}{\Delta x}\] $\frac{\Delta y}{\Delta x}$ is the change in $y$ per unit change in $x$. It is the average rate of change of $y$ with respect to $x$ on the interval $(x,x+\Delta x)$.
When $\Delta x\to 0$, the average rate of change becomes the instantaneous rate of change provided that the limit exists. Thus, the instantaneous rate of change of $y$ with respect to $x$ at $x=x_o$ is given by, \[\lim_{\Delta x\to 0} \left. \frac{\Delta y}{\Delta x}\right|_{x=x_o} =\lim_{\Delta x\to 0}\frac{ƒ(x_o+\Delta x)-ƒ(x_o)}{\Delta x}\] \[\text{or, } \left(\frac{dy}{dx}\right)_{x=x_o}=ƒ'(x_o)=\lim_{\Delta x\to 0}\frac{ƒ(x_o+\Delta x)-ƒ(x_o)}{\Delta x}\]
For example, if $\Delta s$ be the change in distance made by a particle in time $\Delta t$, then $\frac{\Delta s}{\Delta t}$ gives the average rate of change of the distance $s$ (average velocity) and $\lim_{\Delta x\to 0}\frac{\Delta s}{\Delta t}=\frac{ds}{dt}$ is the instantaneous rate of change of $s$ (velocity of the particle in time $t$).
The displacement of the particle varies with time according to the relation $x=-15t^2+20t+30$. Find the velocity and the acceleration of the particle in $\frac{1}{2}$ second. The distance is measured in metre and time in second.
Here, \[x=-15t^2+20t+30\] Differentiating with respect to $t$, \[\frac{dx}{dt}=\frac{d}{dt}(-15t^2+20t+30)\] \[=-30t+20\] \[\frac{d^2x}{dt^2}=\frac{d}{dt}(-30t+20)=-30\]
Hence, velocity of the particle at $t=\frac{1}{2}$ second, \[\left(\frac{dx}{dt}\right)_{t=\frac{1}{2}} =-30×\frac{1}{2}+20=5\text{ ms}^{-1}\]
and, acceleration of the particle at $t=\frac{1}{2}$ second, \[\left(\frac{d^2x}{dt^2}\right)_{t=\frac{1}{2}}=-30 \text{ ms}^{-2}\]
Water is poured into a right circular cylinder of radius $8\text{ cm}$ at the rate of $18\text{ cu.cm/min}$. Find the rate at which the level of water is rising in the cylinder.
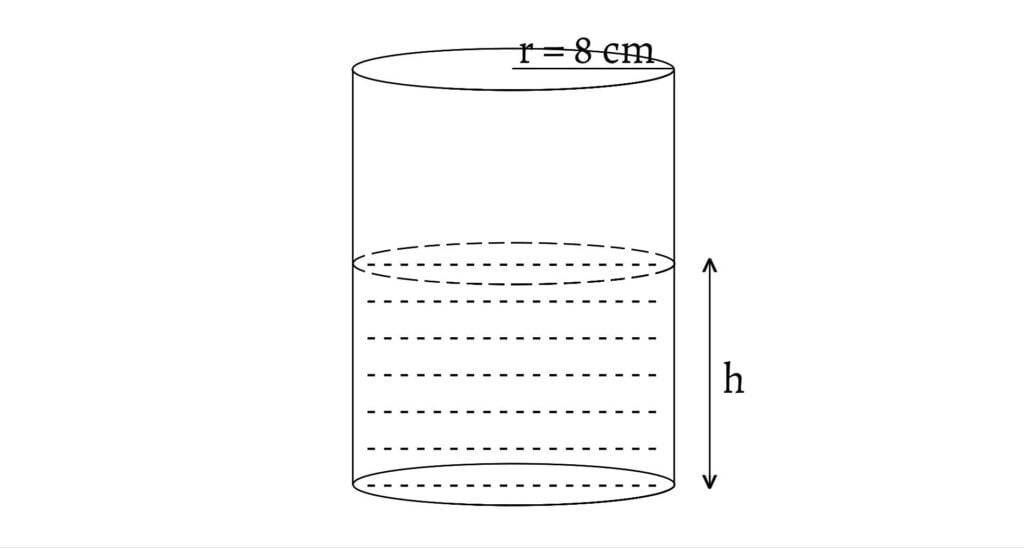
Let $h$ be the water level in the cylinder at any time $t$. Then, \[\frac{dV}{dt}=18 \text{ cm}^3\text{/min}\] Now, \[\text{Volume of oil }(V)=πr^2h\] \[V=64πh\]
Differentiating with respect to $t$, \[\frac{dV}{dt}=64π\frac{dh}{dt}\] \[\frac{dh}{dt}=\frac{18}{64π}\] \[\therefore \frac{dh}{dt}=\frac{9}{32π} \text{ cm/min}\] Hence, the water level is rising in the cylinder at the rate of $\frac{9}{32π}\text{ cm/min}$.
A spherical ball of salt is dissolving in water in such a way that the rate of decrease in volume at any instant is proportional to the surface. Prove that the radius is decreasing at the constant rate.
Let $r$ be the radius of the spherical salt at any time $t$. From question, the rate of decrease in volume is proportional to the surface. So, if $V$ is the volume and $S$ is the surface area of the salt, then, \[-\frac{dV}{dt}∝S\] Negative sign is due to the decreasing volume. \[-\frac{dV}{dt}=kS\] $k$ is a proportionality constant. \[\therefore \frac{dV}{dt}=-kS\]
Now, \[\text{Surface area }(S)=4πr^2\] \[\text{and, Volume }(V)=\frac{4}{3}πr^3\] Differentiating $V$ with respect to $t$, \[\frac{dV}{dt}=4πr^2\frac{dr}{dt}\] \[-kS=4πr^2\frac{dr}{dt}\] \[-k×4πr^2=4πr^2\frac{dr}{dt}\] \[\therefore \frac{dr}{dt}=-k\] Hence, the radius is decreasing at the constant rate.
Water flows into an inverted conical tank at the rate of $42\text{ cm}^3\text{/sec}$. When the depth of the water is $8\text{ cm}$, how fast is the level rising? Assume that the height of the tank is $12\text{ cm}$ and the radius of the top is $6\text{ cm}$.

Let $r$ and $R$ be the radii of water surface and cone respectively. Also, let $h$ and $H$ be the height of water surface and conical tank respectively. Now, \[\Delta PNL \sim \Delta PMA\] \[\therefore \frac{PN}{PM}=\frac{NL}{MA}\] \[\frac{h}{H}=\frac{r}{R}\] \[\frac{h}{12}=\frac{r}{6}\] \[\therefore r=\frac{h}{2}\]
Also, if $V$ is the volume of water, then, \[\frac{dV}{dt}=42\text{ cm}^3\text{/sec}\] Now, \[\text{Volume of water }(V)=\frac{1}{3}πr^2h\]
Putting the value of $r=\frac{h}{2}$ \[V=\frac{1}{3}π\left(\frac{h}{2}\right)^2h\] \[V=\frac{1}{12}πh^3\] Differentiating with respect to $t$, \[\frac{dV}{dt}=\frac{1}{4}πh^2\frac{dh}{dt}\] \[42=\frac{1}{4}πh^2\frac{dh}{dt}\] \[\frac{dh}{dt}=\frac{42×4}{πh^2}\]
When $h=8\text{ cm}$, \[\frac{dh}{dt}=\frac{42×4}{π×8^2}\] \[\frac{dh}{dt}=\frac{21}{8π}\text{ cm/sec}\] Hence, the water level is rising at the rate of $\frac{21}{8π}\text{ cm/sec}$ when $h=8\text{ cm}$.
Two concentric circles are expanding in such a way that the radius of the inner circle is increasing at the rate of $10\text{ cm/sec}$ and that of the outer circle at the rate of $7\text{ cm/sec}$. At a certain time, the radii of the inner and the outer circles are respectively $24\text{ cm}$ and $30\text{ cm}$. At what rate, is the area between the circles increasing or decreasing? How fast?
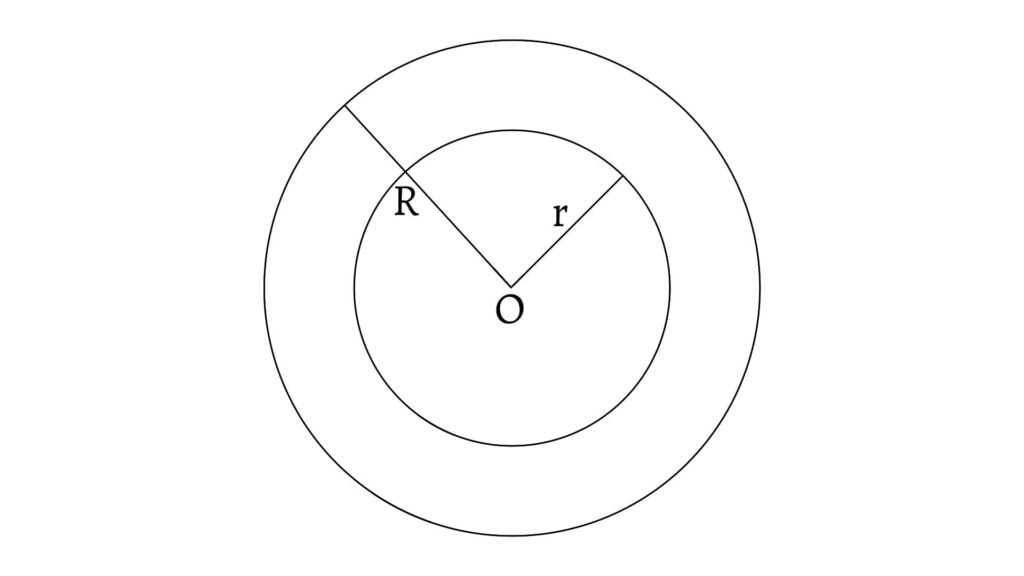
Let $r$ and $R$ be the radii of the inner and outer circles respectively. Then, \[\frac{dr}{dt}=10\text{ cm/sec}\] \[\frac{dR}{dt}=7\text{ cm/sec}\]
Area between the two circles is given by, \[A=πR^2-πr^2\] Differentiating with respect to $t$, \[\frac{dA}{dt}=2πR\frac{dR}{dt}-2πr\frac{dr}{dt}\] \[\frac{dA}{dt}=2πR×7-2πr×10\] \[\frac{dA}{dt}=14πR-20πr\]
When $R=30\text{ cm}$ and $r=24\text{ cm}$, \[\frac{dA}{dt}=14π×30-30π×24\]\[=-60π \text{ cm}^2\text{/sec [decreasing]}\] Hence, the area between the circles is decreasing at the rate of $60π\text{ cm}^2\text{/sec}$.
A $2.5\text{ m}$ ladder leans against a vertical wall. If the top slides downwards at the rate of $12\text{ cm/sec}$, find the speed of the lower end when it is $2\text{ m}$ from the wall.

Let $AC$ be the ladder and $BC$ be the wall. Also, let $AB=x$ and $BC=y$ at any time $t$. Then, \[\frac{dy}{dt}=-12\text{ cm/sec}\] Now, \[AC^2=AB^2+BC^2\] \[2.5^2=x^2+y^2\] Differentiating with respect to $t$, \[0=2x\frac{dx}{dt}+2y\frac{dy}{dt}\] \[0=2x\frac{dx}{dt}+2y×(-12)\] \[2x\frac{dx}{dt}=24y\] \[\therefore \frac{dx}{dt}=\frac{12y}{x}\] When $x=2\text{ m}$, \[2.5^2=2^2+y^2\Rightarrow y=1.5\text{ m}\] \[\therefore \frac{dx}{dt}=\frac{12×1.5}{2}=9\text{ cm/sec}\] Hence, the speed of the lower end is $9\text{ cm/sec}$ when it is $2\text{ m}$ from the wall.
A man of height $1.5\text{ m}$ walks away from a lamp post of height $4.5\text{ m}$ at the rate of $20\text{ cm/sec}$. How fast is the shadow lengthening when the man is $42\text{ cm}$ from the post?
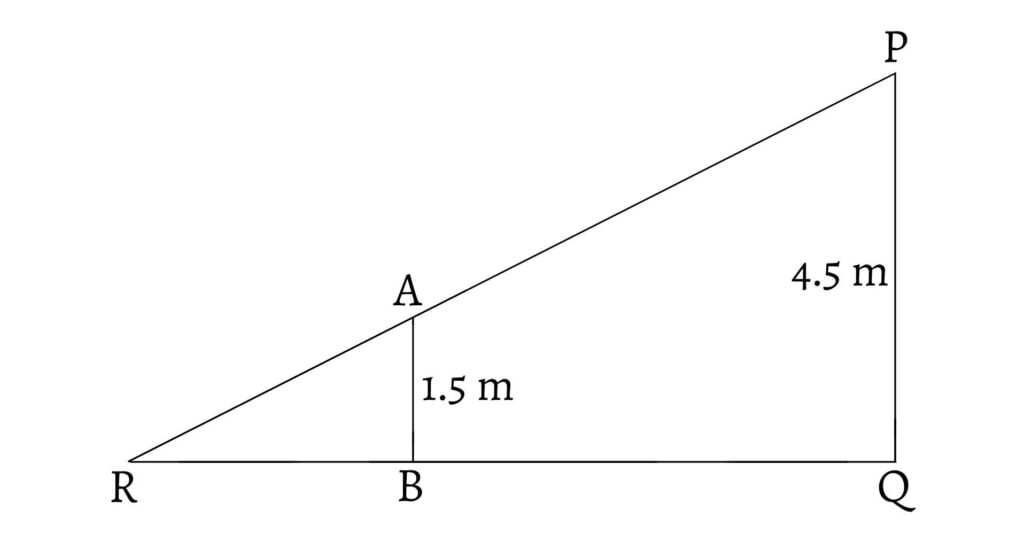
Let $PQ=4.5 \text{ m}$ and $AB=1.5\text{ m}$ be the lamp post and man respectively. Also, let $RB=x$ which is the shadow and $BQ=y$ at any time $t$. Then, \[\frac{dy}{dt}=20\text{ cm/sec}\] Now, \[\Delta RAB\sim\Delta RPQ\] \[\therefore \frac{RB}{RQ}=\frac{AB}{PQ}\] \[\frac{x}{x+y}=\frac{1.5}{4.5}\] \[\frac{x}{x+y}=\frac{1}{3}\] \[3x=x+y\] \[y=2x\] Differentiating with respect to $t$, \[\frac{dy}{dt}=2\frac{dx}{dt}\] \[20=2\frac{dx}{dt}\] \[\therefore \frac{dx}{dt}=10\text{ cm/sec}\] Hence, the shadow is lengthening at the rate of $10\text{ cm/sec}$.
