Ellipse

An ellipse is the locus of a point in a plane such that the sum of the distances of the point from two fixed points is constant.

The two fixed points $S$ and $S’$ are the foci of the ellipse and the midpoint between them is the centre of the ellipse. In the figure given above, we take the origin $O$ as the centre of the ellipse.

The line through the two foci is known as the major axis of the ellipse. And the line perpendicular to the major axis is known as its minor axis. The intersection of the ellipse and the major axis gives two points $A$ and $A’$ which are called vertices.

An ellipse can also be defined as the locus of a point in a plane such that the ratio (called eccentricity) of its distance from a fixed point (called the focus) to its distance from a fixed straight line (called the directrix) is constant. The eccentricity (denoted by $e$) is any number between $0$ and $1$.

Standard Equation

Let the centre of the ellipse be at the origin $O$ and the major axis along the x-axis. We call this the ellipse in the standard position. Let the two foci be $S(c,0)$ and $S(-c,0)$ where $c$ is a positive constant.

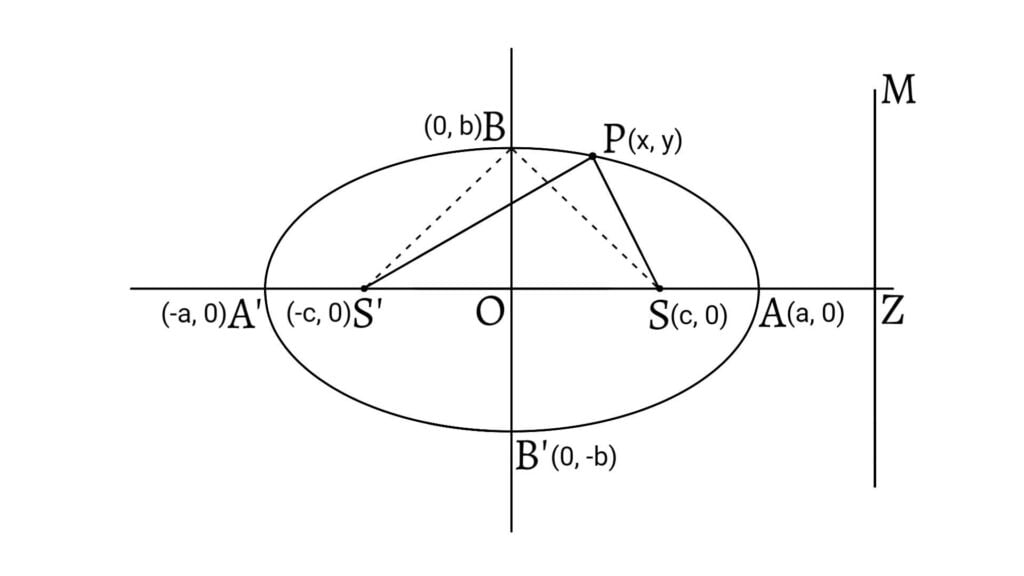
Take any point $P(x,y)$ on the ellipse, then \[PS+PS’=\text{Constant}\] \[\text{Let}\;PS+PS’=2a\] \[\text{Obviously,}\;2s>2a\Rightarrow a>c\] Using distance formula, \[\sqrt{(x+c)^2+y^2}+\sqrt{(x-c)^2+y^2}=2a\] \[(x+c)^2+y^2=4a^2-4a\sqrt{(x-c)^2+y^2}+(x-c)^2+y^2\] \[4cx-4a^2=-4a\sqrt{(x-c)^2+y^2}\] \[(cx-a^2)^2=a^2[(x-c)^2+y^2]\] \[c^2x^2-2a^2cx+a^4=a^2[x^2-2cx+c^2+y^2]\] \[a^2(a^2-c^2)=(a^2-c^2)x^2+a^2y^2\] \[\therefore\frac{x^2}{a^2}+\frac{y^2}{a^2-c^2}=1\]

$a^2-c^2$ is a positive constant. Let $b^2=a^2-c^2$. Then the equation of the ellipse becomes \[\frac{x^2}{a^2}+\frac{y^2}{b^2}=1\] The vertices of the ellipse are $A(a,0)$ and $A'(-a,0)$. The distance between them, $2a$, is called the length of the major axis. The ellipse intersects the y-axis at points $B(0,b)$ and $B(0,-b)$. The distance between them, $2b$, is called the length of the minor axis.

Let $ZM$ be the directrix of the ellipse. Then, the distance of a point on the ellipse from the focus and the distance of that point from the directrix bears a constant ratio. This constant ratio is called eccentricity denoted by $e$. Therefore \[e=\frac{AS}{AZ}\;\text{and}\;e=\frac{A’S}{A’Z}\] \[\therefore A’S-AS=e(A’Z-AZ)\] \[(A’O+OS)-(OA-OS)=e\,AA’\] \[2OS=2ae\] \[\therefore OS=ae\;\text{i.e.}\;c=ae\]

Hence, we have \[e=\frac{c}{a}=\frac{\sqrt{a^2-b^2}}{a}=\sqrt{1-\frac{b^2}{a^2}}\] Since $c<a$, $e$ is always between $0$ and $1$. The coordinates of the foci are $(\pm ae,0)$. Also, \[AS+A’S=e(AZ+A’Z)\] \[2a=2eOZ\] \[\therefore OZ=\frac{a}{e}\] Hence, the equation of the directrix is $x=\pm\frac{a}{e}$.

If in the equation of an ellipse, \[\frac{x^2}{a^2}+\frac{y^2}{b^2}=1\] $b>a>0$, then the major axis will be along the y-axis and the minor axis along the x-axis. In this case, $b^2=a^2+c^2$ and \[e=\frac{c}{b}=\frac{\sqrt{b^2-a^2}}{b}=\sqrt{1-\frac{a^2}{b^2}}\] Also, the equation of the directrix will be $y=\pm\frac{b}{e}$.

Equation of an Ellipse whose Centre is not at the Origin [Centre at $(h,k)$]

Let the centre of the ellipse be at $(h,k)$. Then the equation of the ellipse is given by \[\frac{(x-h)^2}{a^2}+\frac{(y-k)^2}{b^2}=1\;(a>b>0)\]

The major axis and minor axis are parallel to x-axis and y-axis respectively. The vertex of the ellipse is $(h\pm a,k)$ and focus is $(h\pm ae,k)$. Eccentricity $(e)$ is given by \[e=\sqrt{1-\frac{b^2}{a^2}}\] And, the equation of the directrix is $x=h\pm\frac{a}{e}$.

Similarly, If in the equation \[\frac{(x-h)^2}{a^2}+\frac{(y-h)^2}{b^2}=1\] $b>a>0$, then the major axis and minor axis will be parallel to y-axis and x-axis respectively. The vertex will be $(h,k\pm b)$ and focus will be $(h,k\pm be)$. Eccentricity $(e)$ is given by \[e=\sqrt{1-\frac{a^2}{b^2}}\] And, the equation of the directrix is $y=k\pm\frac{b}{e}$.

---

---