Let the two straight lines represented by the equation \[ax^2+2hxy+by^2=0\] be $y=m_1x$ and $y=m_2x$ so that \[m_1+m_2=-\frac{2h}{b}\text{ and }m_1m_2=\frac{a}{b}\] Let $OA$ and $OB$ be the two lines as in the figure and let $OC$ and $OD$ be the bisectors of the angles between them.
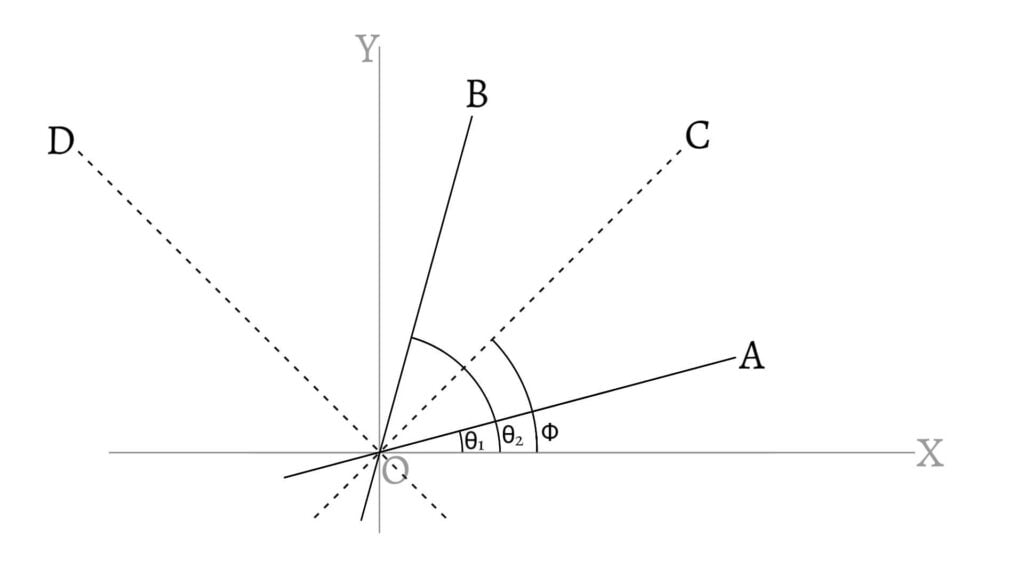
If $OA$ and $OB$ make angles $\theta_1$ and $\theta_2$ with $OX$, then $\tan\theta_1=m_1$ and $\tan\theta_2=m_2$. Let $OC$ make an angle $\phi$ with $OX$. From the figure, we have \[\angle XOC=\angle XOA+\angle AOC\] \[\phi=\theta_1+\frac{1}{2}(\theta_2-\theta_1)=\frac{1}{2}(\theta_1+\theta_2)\] \[2\phi=\theta_1+\theta_2\] \[\tan 2\phi=\tan(\theta_1+\theta_2)\] \[\text{i.e. }\tan(2\angle XOC)=\tan 2\phi=\tan(\theta_1+\theta_2)\]
Also, \[\angle XOD=\angle XOC+90°=\phi+90°\] \[\text{and }\tan(2\angle XOD)=\tan(2\phi+180°)=\tan 2\phi\] Hence if $(x,y)$ be any point in $OC$, or $OD$, we have $\tan\angle XOC$ or $\tan\angle XOD=\frac{y}{x}$, and $\tan 2\angle XOD=\tan 2\angle XOC=\tan 2\phi$.
\[\text{But }\tan 2\phi=\tan(\theta_1+\theta_2)\] \[\therefore\frac{2\tan\phi}{1-\tan^2\phi}=\frac{\tan\theta_1+\tan \theta_2}{1-\tan\theta_1\tan\theta_2}\] \[\frac{2\frac{y}{x}}{1-\left(\frac{y}{x}\right)^2}=\frac{m_1+m_2}{1-m_1m_2}\] \[\frac{2\frac{y}{x}}{1-\frac{y^2}{x^2}}=\frac{-\frac{2h}{b}}{1-\frac{a}{b}}\] \[\therefore h(x^2-y^2)=(a-b)xy\] \[\text{or, }\frac{xy}{x^2-y^2}=\frac{h}{a-b}\] Since this is true for any point $(x,y)$ in $OC$ or $CD$, it represents the two bisectors.
Alternative Method
Here, \[ax^2+2hxy+by^2=0\] Let \[\begin{array}{c}y=m_1x &\text{and}& y=m_2x\\ \text{or, } m_1x-y=0 &\text{and}& m_2x-y=0\end{array}\] be the two lines represented by $ax^2+2hxy+by^2=0$. Then, \[m_1+m_2=-\frac{2h}{b}\text{ and }m_1m_2=\frac{a}{b}\] Now, the equations of the bisectors of the angles between above lines are \[\frac{m_1x-y}{\sqrt{m_1^2+1}}=\pm\frac{m_2x-y}{\sqrt{m_2^2+1}}\]
\[(m_1x-y)^2(1+m_2^2)=(m_2x-y)^2(1+m_1^2)\] \[\text{or, }(m_1^2x^2-2m_1xy+y^2)(1+m_2^2)\]\[=(m_2^2x^2-2m_2xy+y^2)(1+m_1^2)\] \[\text{or, }m_1^2(1+m_2^2)x^2-2m_1(1+m_2^2)xy+(1+m_2^2)y^2\] \[=m_2^2(1+m_1^2)x^2-2m_2(1+m_1^2)xy+(1+m_1^2)y^2\] \[\text{or, }[m_1^2(1+m_2^2)-m_2^2(1+m_1^2)]x^2+[(1+m_2^2)\]\[-(1+m_1^2)]y^2=2[m_1(1+m_2^2)-m_2(1+m_1^2)]xy\] \[\text{or, }(m_1^2+m_1^2m_2^2-m_2^2-m_2^2m_1^2)x^2-(m_1^2-m_2^2)y^2\] \[=2[m_1+m_1m_2^2-m_2-m_1^2m_2]xy\] \[\text{or, }(m_1^2-m_2^2)x^2-(m_1^2-m_2^2)y^2\] \[=2[m_1-m_2-m_1m_2(m_1-m_2)]xy\]
\[\text{or, }(m_1^2-m_2^2)(x^2-y^2)=2(m_1-m_2)(1-m_1m_2)xy\] \[\text{or, }(m_1+m_2)(x^2-y^2)=2(1-m_1m_2)xy\] \[\text{or, }\frac{m_1+m_2}{1-m_1m_2}=\frac{2xy}{x^2-y^2}\] \[\text{or, }\frac{-\frac{2h}{b}}{1-\frac{a}{b}}=\frac{2xy}{x^2-y^2}\] \[\text{or, }\frac{-2h}{b-a}=\frac{2xy}{x^2-y^2}\] \[\text{or, }\frac{2h}{a-b}=\frac{2xy}{x^2-y^2}\] \[\therefore\frac{xy}{x^2-y^2}=\frac{h}{a-b}\] which is the required equation.
Find the equations of the bisectors of the angles between the line pair $3x^2-15xy+2y^2=0$.
Given line pair is, \[3x^2-15xy+2y^2=0\] \[\begin{array}{c}\therefore a=3, & 2h=-15 & \text{and} & b=2 \\ a=3, & h=-\frac{15}{2} &\text{and}& b=2\end{array}\] The equations of the bisectors are, \[\frac{xy}{x^2-y^2}=\frac{h}{a-b}\] \[\frac{xy}{x^2-y^2}=\frac{-\frac{15}{2}}{3+2}\] \[\frac{xy}{x^2+y^2}=-\frac{3}{2}\] \[2xy=-3x^2+3y^2\] \[\therefore 3x^2+2xy-3y^2=0\]
If the line pairs $ax^2+2hxy+by^2=0$ and $a’x^2+2h’xy+b’y^2=0$ have the same bisectors, prove that $h(a’-b’)=h'(a-b)$.
Here, \[ax^2+2hxy+by^2=0\text{ __(1)}\] The equations of the bisectors of the angles between the line pair $\text{(1)}$ are \[\frac{xy}{x^2-y^2}=\frac{h}{a-b}\] Also, \[a’x^2+2h’xy+b’y^2=0\text{ __(2)}\] The equations of the bisectors of the angles between the line pair $\text{(2)}$ are \[\frac{xy}{x^2-y^2}=\frac{h’}{a’-b’}\] Since the bisectors are same, \[\frac{h}{a-b}=\frac{h’}{a’-b’}\] \[\therefore h(a’-b’)=h'(a-b)\]
If the pair of lines $x^2-2pxy-y^2=0$ and $x^2-2qxy-y^2=0$ be such that each pair bisects the angles between the other pair, prove that $pq=-1$.
The pair of bisectors of the angles between the line pair $x^2-2pxy-y^2=0$ is given by, \[\frac{xy}{x^2-y^2}=\frac{-p}{1-(-1)}\] \[2xy=-px^2+py^2\] \[\therefore px^2+2xy-py^2=0\] According to the question, the pair of bisectors is given by $x^2-2qxy-y^2=0$. Hence, $px^2+2xy-py^2=0$ and $x^2-2qxy-y^2=0$ must represent the same pair of lines. \[\therefore\frac{p}{1}=\frac{2}{-2q}=\frac{-p}{-1}\] \[\therefore pq=-1\]
