The inability of a lens to bring the light of different colors to focus at a single point is called axial or longitudinal chromatic aberration.
A lens can be considered as the combination of a number of prisms placed one above the other. Due to its prismatic action, when a beam of light is incident on the lens parallel to its principal axis, it gets splits up into its constituent colors. The various colors are brought into focus at different points as the focal length of the lens is given by,
\[\frac{1}{f}=(μ-1)\left(\frac{1}{R_1}+\frac{1}{R_2}\right)\]
Hence, the focal length depends upon the refractive index of the material which further depends upon the color of light.
The refractive index of a glass is greater for violet light than that for red light i.e. $μ_v>μ_r$. Hence, the focal length of the lens for the red light is greater than that for violet light. The violet ray of the light gets focused at a point $F_v$ which is closer to the lens and the red ray of the light gets focused at a point $F_r$ which is a little away from the lens. The other colors are focused on the principal axis between points $F_v$ and $F_r$.
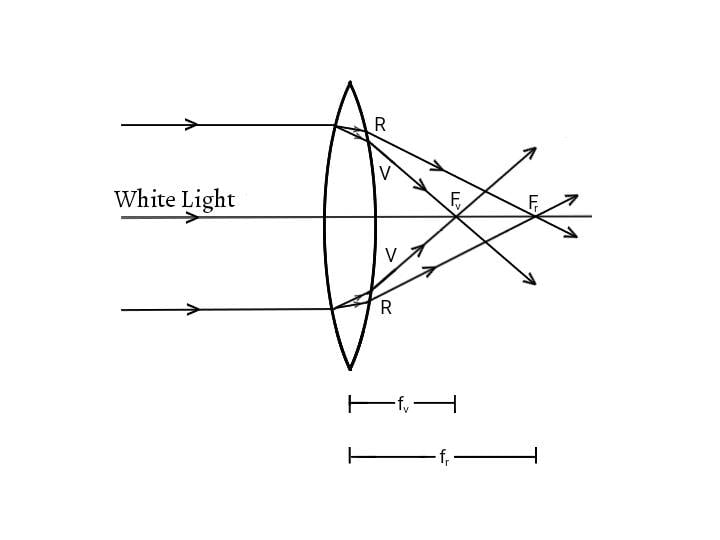
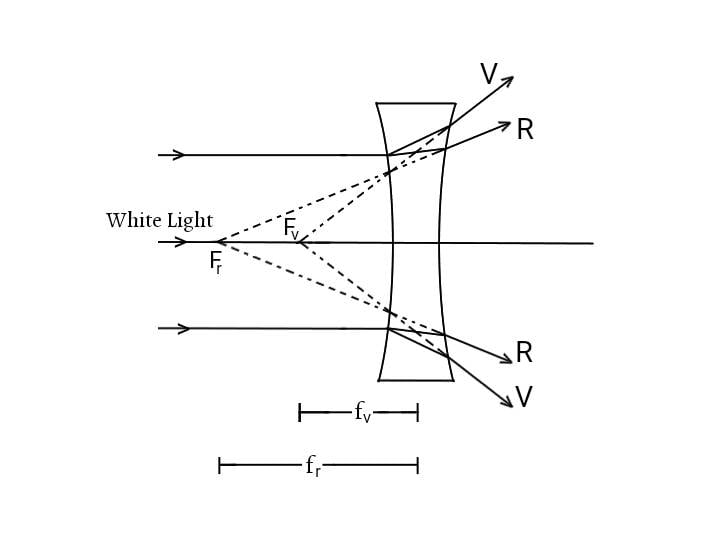
If $f_r$ and $f_v$ are the focal lengths of the lens for violet and red light then the difference $f_r-f_v$ gives the measure of the longitudinal chromatic aberration.
\[\text{Longitudinal Chromatic Aberration}=f_r-f_v\]
Expression for Longitudinal Chromatic Aberration
The focal length of the lens for mean light is given by, \[\frac{1}{f}=(μ-1)\left(\frac{1}{R_1}+\frac{1}{R_2}\right)\]
Where, $R_1$ and $R_2$ are the radii of curvature of the two surfaces of the lens, and $μ$ is the refractive index of the material of the lens for the mean light. \[\left(\frac{1}{R_1}+\frac{1}{R_2}\right)=\frac{1}{f(μ-1)}\text{ ____(1)}\]
Let $(μ_v, μ_r)$ and $(f_v, f_r)$ be the refractive index of the material of the lens and the focal length of the lens for violet and red light respectively.
Focal length of the lens for violet light is given by, \[\frac{1}{f_v}=(μ_v-1)\left(\frac{1}{R_1}+\frac{1}{R_2}\right)\text{ ____(2)}\]
Focal length of the lens for red light is given by, \[\frac{1}{f_r}=(μ_r-1)\left(\frac{1}{R_1}+\frac{1}{R_2}\right)\text{ ____(3)}\]
Substituting the value of $\left(\frac{1}{R_1}+\frac{1}{R_2}\right)$ in equation $(2)$ and $(3)$,
\[\frac{1}{f_v}=\frac{μ_v-1}{f(μ-1)} \text{ ____(4)}\]
\[\text{and, }\frac{1}{f_r}=\frac{μ_r-1}{f(μ-1)} \text{ ____(5)}\]
Subtracting equation $(5)$ from $(4)$,
\[\frac{1}{f_v}-\frac{1}{f_r}=\frac{μ_v-1}{f(μ-1)}- \frac{μ_r-1}{f(μ-1)}\]
\[\frac{f_r-f_v}{f_vf_r}=\frac{(μ_v-1)-(μ_r-1)}{f(μ-1)}\]
\[f_r-f_v=\frac{μ_v-μ_r}{f(μ-1)}f_vf_r\text{ ____(6)}\]
The focal length of the lens for mean light can be taken as the geometric mean of the focal lengths for violet and red light i.e. \[f=\sqrt{f_vf_r}\] \[f_rf_v=f^2 \text{ ____(7)}\]
Also, the dispersive power of the material of the lens is given by, \[ω=\frac{μ_v-μ_r}{f(μ-1)} \text{ ____(8)}\]
From equations $(6)$, $(7)$ and $(8)$, \[f_r-f_v=\frac{ω}{f}f^2\] \[f_r-f_v=ωf\]
Chromatic Aberration $=$ Dispersive Power $×$ Mean Focal Length
The dispersive power $ω$ is always positive, whereas $f$ is positive for convex lens and negative for concave lens. Therefore, chromatic aberration is positive for convex lens and it is negative for concave lens.
More on Dispersion
