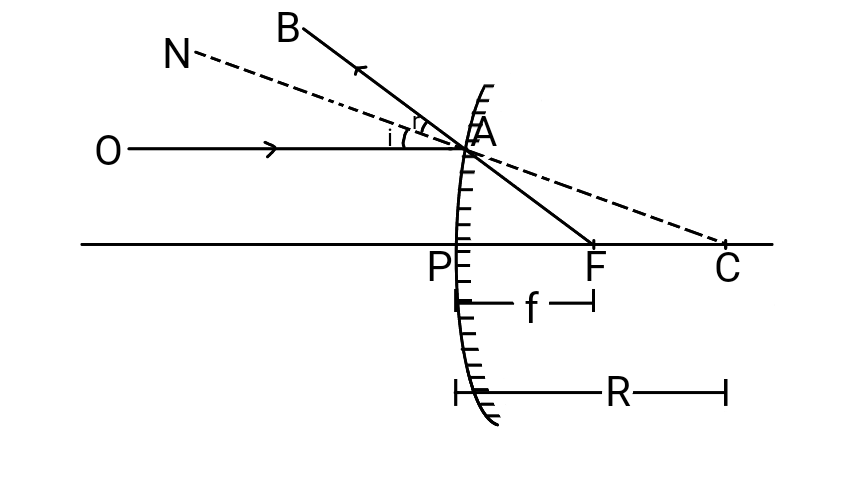
For Concave Mirror
Let the concave spherical mirror has focal length $f$ and radius of curvature $R$. Let $P$, $F$ and $C$ be its pole, focus and centre of curvature respectively. Consider a ray of light $OA$ is incident on the mirror which is parallel to the principal axis of the mirror. Join $AC$. Then, $AC$ is normal to the mirror at point $A$. Let the ray of light gets reflected along $AB$ cutting the pricipal axis at point $F$. Let $i$ be the angle of incidence and $r$ be the angle of reflection.

Then, \[\text{angle of incidence} = \text{angle of reflection}\] \[∠OAC=∠CAF\text{ __(1)}\] also, \[∠OAC= ∠ACF\text{ __(2) [Being alternate angles]}\]
From $(1)$ and $(2)$, \[∠CAF=∠ACF\] Thus, $ΔACF$ is an isosceles triangle. \[∴CF=AF\text{ __(3)}\] Now, \[CP=CF+FP\text{ __(4)}\]
From $(3)$ and $(4)$, \[CP=AF+FP\text{ __(5)}\] The points $A$ and $P$ lies very close to each other because the aperture of the mirror is small. Thus, \[\text{when }A\to P\text{,}\] \[AF ≈FP\]
Therefore, equation $(4)$ becomes, \[CP=FP+FP\] \[CP=2FP\] \[FP=\frac{1}{2}CP\] \[∴f=\frac{1}{2}R\] This is the relation between focal length and radius of curvature for concave mirror.
[Also see: Lateral Shift]
For Convex Mirror
In the similar way, let the convex spherical mirror has focal length $f$ and radius of curvature $R$. Let $P$, $F$ and $C$ be its pole, focus and centre of curvature respectively. Consider a ray of light $OA$ is incident on the mirror which is parallel to the principal axis of the mirror. Then, the ray of light gets reflected along $AB$. Join $AC$ and produce outward. Then, $AC$ is normal to the mirror at point $A$. Let $i$ be the angle of incidence and $r$ be the angle of reflection.
Then, \[\text{angle of incidence} = \text{angle of reflection}\] \[∠OAN= ∠BAN\text{ __(a)}\]
Now, \[∠FAC=∠BAN\text{ __(b) [Being vertically opp. angles]}\] \[∠FCA=∠OAN\text{ __(c) [Being corresponding angles]}\]
From $(a)$, $(b)$ and $(c)$, \[∠FAC=∠FCA\] Thus, $ΔACF$ is an isosceles triangle. \[∴CF=AF\text{ __(3)}\] Now, \[CP=CF+FP\text{ __(4)}\] From $(3)$ and $(4)$, \[CP=AF+FP\text{ __(5)}\]
Since the aperture of the mirror is small, \[\text{when }A\to P\text{,}\] \[AF ≈FP\] Therefore, equation $(4)$ becomes, \[CP=FP+FP\] \[CP=2FP\] \[FP=\frac{1}{2}CP\] \[∴f=\frac{1}{2}R\] This is the relation between focal length and radius of curvature for convex mirror.
[Also See: Dispersion]
