When two parallel conductors carrying current are close together, they exert forces to each other. The magnetic fields developed due to both conductors interact which causes the force acting between them. The nature of this force may be either attractive or repulsive depending on the direction of the currents in the conductor. If currents are in same direction, they are called like currents and the conductors attract each other while if the currents are in the opposite directions, they are called unlike currents and the conductors repel each other. Let us discuss the following two cases:
Force between two parallel conductors carrying currents in the same direction
Consider two infinitely long parallel conductors $1$ and $2$ separated by a distance $r$ and carrying currents $I_1$ and $I_2$ respectively in same direction.
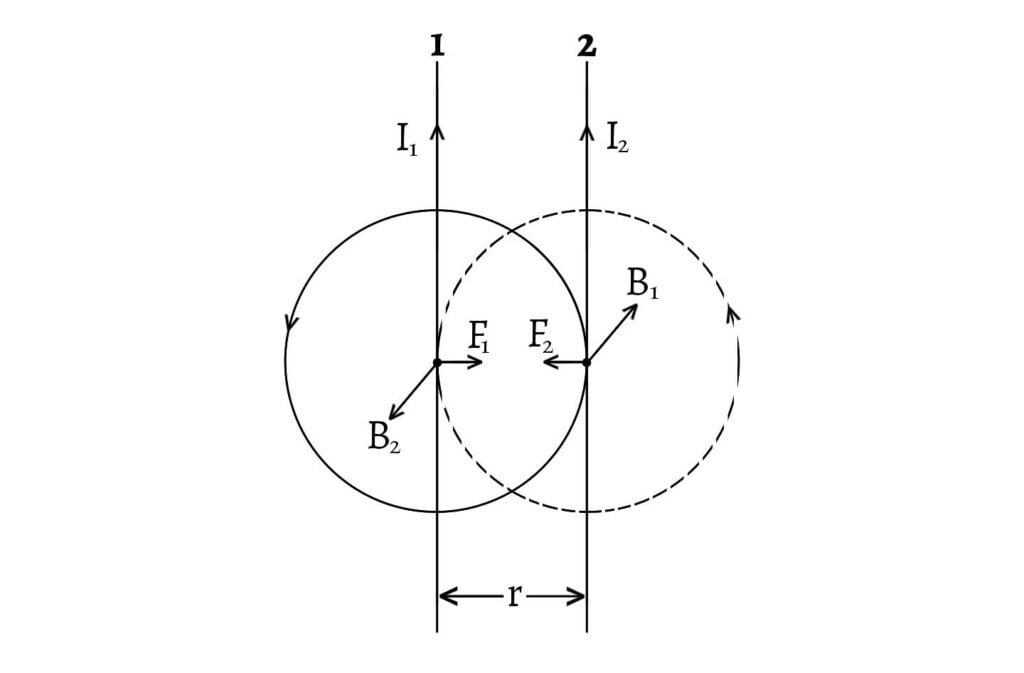
The magnetic field $B_1$ is developed due to current $I_1$ and $B_2$ due to current $I_2$. The wire $2$ is placed inside the magnetic field $B_1$ and the wire $1$ inside the field $B_2$.
The magnetic field $B_1$ is given by, \[B_1=\frac{\mu_oI_1}{2πr}\]
According to right hand thumb rule, the direction of $B_1$ is perpendicular to wire $2$. The magnetic force $F_2$ acting on wire $2$ for a length $l$ is given by,
\[F_2=B_1I_2l\sin90°\]
\[F_2=\frac{\mu_oI_1I_2l}{2πr}\]
According to Fleming’s left hand rule, the direction of force $F_2$ is perpendicular to length of wire $2$ acting towards wire $1$.
Also, the magnetic field $B_2$ is given by, \[B_2=\frac{\mu_oI_2}{2πr}\]
According to right hand thumb rule, the direction of $B_2$ is perpendicular to wire $1$. The magnetic force $F_1$ acting on wire $1$ for the length $l$ is given by,
\[F_2=B_2I_1l\sin90°\]
\[F_1=\frac{\mu_oI_1I_2l}{2πr}\]
According to Fleming’s left hand rule, the direction of $F_1$ is perpendicular to the length of wire $1$ and directed towards the wire $2$.
Here, the forces $F_1$ and $F_2$ have same magnitude but towards each other which is attractive force.
\[\text{i.e. }F_1=F_2=f\text{ (say)}\]
\[\therefore f=\frac{\mu_oI_1I_2l}{2πr}\]
Hence, the attractive force per unit length acting between two infinitely long parallel conductors is given by,
\[\frac{f}{l}=\frac{\mu_oI_1I_2}{2πr}\]
\[\therefore F=\frac{\mu_oI_1I_2}{2πr}\]
According to Newton’s third law of motion, these forces are action and reaction for each other. Hence, it is concluded that the wires carrying currents in same direction i.e. like currents attract each other with the same force.
In a solenoid, the adjacent turns carry parallel currents in the same direction. So the turns experience attractive force towards each other, causing a compressive stress along the solenoid axis. Thus, when current is passed through a solenoid, it contracts.
Force between two parallel conductors carrying current in the opposite direction
Let us consider two infinitely long parallel conductors $1$ and $2$ separated by a distance $r$ and carrying currents $I_1$ and $I_2$ respectively in opposite directions.
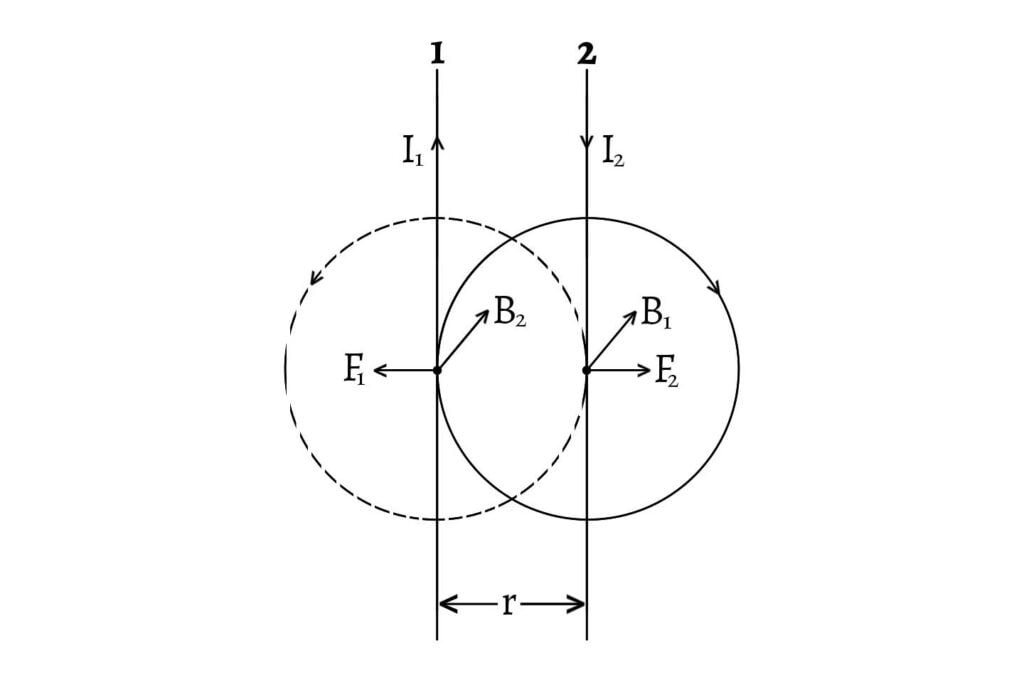
The magnetic field $B_1$ is developed due to current $I_1$ and $B_2$ due to current $I_2$. The wires are placed in such a way that one wire is on region of magnetic field caused by other wire.
The magnetic field $B_1$ is given by,
\[B_1=\frac{\mu_oI_1}{2πr}\]
According to right hand thumb rule, the direction of $B_1$ is perpendicular to the wire $2$. The magnetic force $F_2$ acting on wire $2$ for a length $l$ is given by,
\[F_2=B_1I_2l\sin90°\]
\[F_2=\frac{\mu_oI_1I_2l}{2πr}\]
According to Fleming’s left hand rule, the direction of $F_2$ is perpendicular to wire $2$ and is directed towards the opposite direction to the wire $1$.
The magnetic field $B_2$ is given by,
\[B_2=\frac{\mu_oI_2}{2πr}\]
According to right hand thumb rule, the direction of $B_2$ is perpendicular to the wire $1$. The magnetic force $F_1$ acting on wire $1$ for the length $l$ is given by,
\[F_1=B_2I_1l\sin90°\]
\[F_1=\frac{\mu_oI_1I_2}{2πr}\]
According to Fleming’s left hand rule, the direction of $F_1$ is perpendicular to wire $1$ and is directed towards the direction opposite to the wire $2$.
Here, the forces $F_1$ and $F_2$ have same magnitude but they are directed away from each other which is repulsive force. \[\text{i.e. }F_1=F_2=f \text{ (say)}\] \[\therefore f=\frac{\mu_oI_1I_2l}{2πr}\]
Hence, the repulsive force per unit length acting between two infinitely long parallel conductors is given by,
\[\frac{f}{l}=\frac{\mu_oI_1I_2}{2πr}\]
\[\therefore F=\frac{\mu_oI_1I_2}{2πr}\]
According to Newton’s third law of motion, these forces are action and reaction for each other. Hence, it is concluded the wires carrying the currents in opposite directions i.e. unlike currents repel each other with same force.
Hence, we have the following relation for attractive or repulsive force per unit length between two long parallel straight current carrying conductors,
\[F=\frac{\mu_o}{2r}\frac{I_1I_2}{r}\]
From this relation, we can see that the force is directly proportional to the product of the currents in the conductors and inversely proportional to the distance by which the two conductors are separated.
Force between non parallel conductors carrying currents
The force between two non parallel currents for different configurations of the conductors are shown in the following figure.
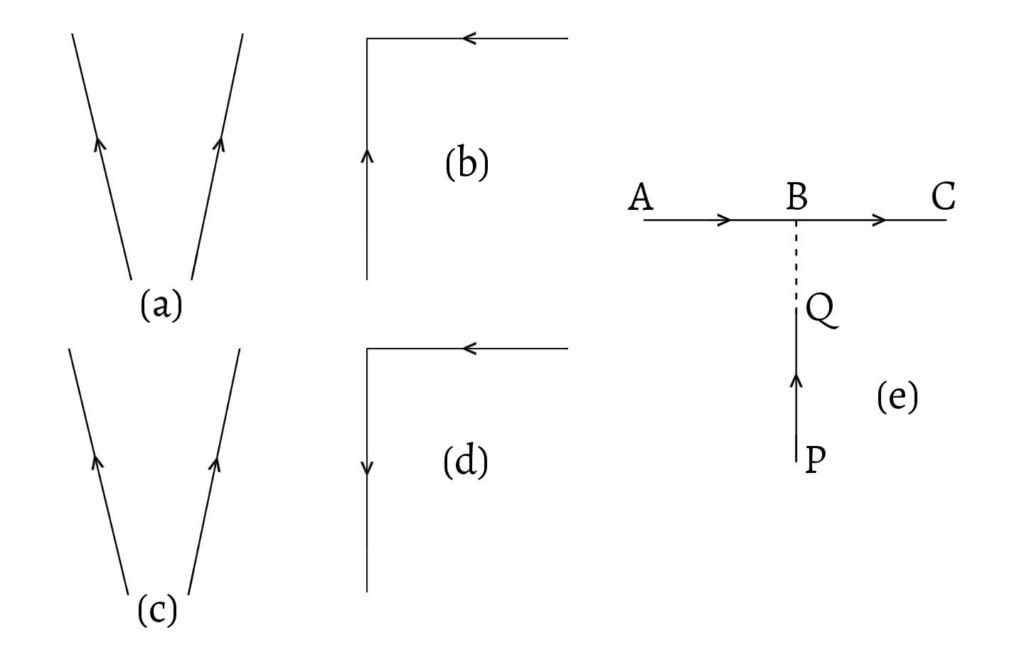
When two conductors are arranged as shown in figure (a) and figure (b), they attract each other and when they are arranged as in figure (c) and (d), they repel each other. When they are arranged as shown in figure (e), there is attraction between the parts AB and PQ while there is repulsion between parts BC and PQ od the conductors.
Hence, two non parallel currents attract each other if both currents diverge from a point or converge to a point and they repel each other if one current approaches a point and the other current recedes away from the point.
Definition of one Ampere on the basis of the force between the current in parallel conductors
The mutual force of attraction or repulsion per unit length between two long parallel conductors is given by,
\[F=\frac{\mu_oI_1I_2}{2πr}\]
Putting $I_1=I_2=1 \text{ A}$ and $r=1 \text{ m}$, we get,
\[F=\frac{4π×10^{-7}×1×1}{2π×1}=2×10^{-7} \text{ Nm}^{-1}\]
Thus, the current is said to be of one Ampere if it flows through two infinitely log parallel conductors separated by a distance of 1 m in vacuum so that it can produce force per unit length of magnitude $2×10^{-7} \text{ Nm}^{-1}$ between them.
