The force experienced by a charged particle in electric or magnetic or both fields is called Lorentz force (force on a moving charge). It is of two types; electric Lorentz force and magnetic Lorentz force.
Electric Lorentz Force
The force experienced by a charged particle in an electric field is called electric Lorentz force. If $\overrightarrow{E}$ is the electric intensity at a certain point in space then the electric Lorentz force $\overrightarrow{F}$ experienced by a charged particle at that point is given by,
\[\overrightarrow{F}=q\overrightarrow{E}\] and its magnitude is given by \[F=qE\]
A charged particle in an electric field experiences electric force whether it is in motion or at rest.
Magnetic Lorentz Force
When an electric charge moves through a magnetic field, the field exerts a force on that charge. Consider a charge $+q$ moving with velocity $v$ in a plane $XZ$ by making an angle $\theta$ with magnetic field $B$ along $\text{X-axis}$.
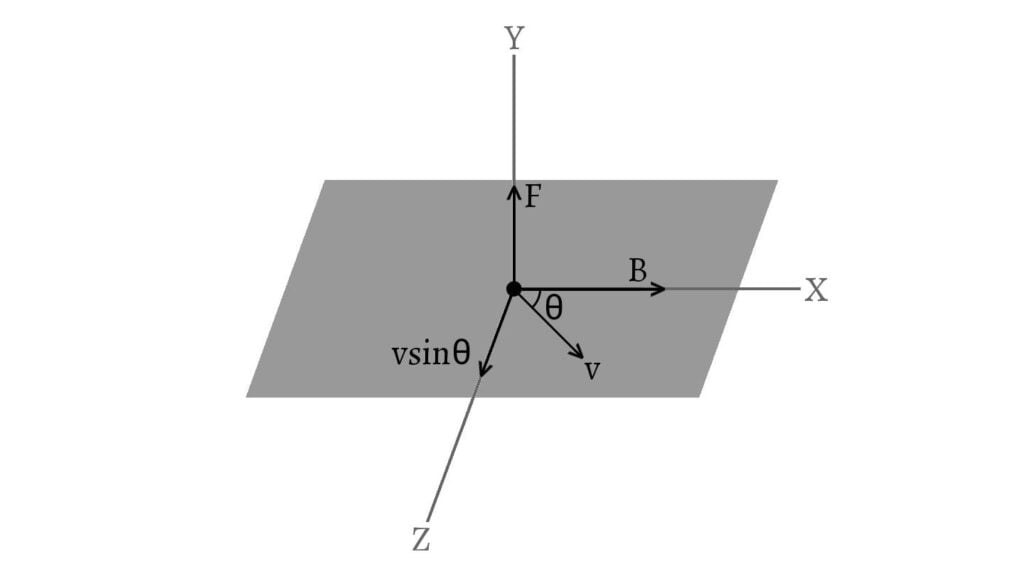
In such case, the charge experiences a magnetic force along $\text{Y-axis}$, is perpendicular to the plane of $v$ and $B$. Experimentally, it is found that magnitude of magnetic force $F$ is:
- directly proportional to the magnitude of the charge \[F∝q\]
- directly proportional to the velocity of the charge \[F∝v\]
- directly proportional to the strength of magnetic field \[F∝B\]
- directly proportional to the sine of the angle between $v$ and $B$ \[F∝\sin\theta\]
Combining these equations, we get, \[F∝qvB\sin\theta\] \[F=kqvB\sin\theta\]
Where $k$ is the proportionality constant whose value is $1$ in SI units. Therefore, we have, \[F=qvB\sin\theta\]
In vector form, \[\overrightarrow{F}=q(\overrightarrow{v}×\overrightarrow{B})\]
The force $\overrightarrow{F}$ is the vector perpendicular to $\overrightarrow{v}$ and $\overrightarrow{B}$. This force is called magnetic Lorentz force.
The force $F$ acts perpendicular to $v$ and $B$, so $F$ is a deflecting force. It does not change the magnitude of $v$, but changes the direction of motion of the charge when it enters the field $B$. Since $F$ is perpendicular to the displacement of charge, no work is done by $F$. So, no energy is gained by the charge when it enters the field $B$ and $F$ acts on it.
Special Cases
- If $v=0$, $F=0$ i.e. a charge at rest does not experience any magnetic force.
- If $\theta=0°$ or $180°$, $F=0$ i.e. the charge does not experience any magnetic force if $v$ is either parallel or antiparallel to the direction of $B$. If no force acts on a particle, there will be no acceleration in the particle. The particle having no acceleration indicates that its velocity does not change. The velocity is a vector quantity and it does not change so its magnitude and direction both do not change. If magnitude of the velocity does not change, the kinetic energy of the particle does not change, if kinetic energy is not changed, then work done by this force is also zero [Work Energy Theorem]. The direction of the particle is not changed so there is no deflection of the particle in the uniform magnetic field. Hence, the nature of the path of the particle in a uniform magnetic field is a straight line.
- If $\theta=90°$, $F=F_{\text{max}}$ i.e. the charged particle experiences maximum magnetic force when it is perpendicular to the direction of magnetic field.
- If the velocity of a charged particle is neither parallel, antiparallel nor normal to the magnetic field, it gets deflected and follows a spiral path in the magnetic field.
Lorentz Force in both Electric and Magnetic Field
If a charged particle $q$ is placed in both electric and magnetic fields, then the electric Lorentz force $\overrightarrow{F_e}$ and the magnetic Lorentz force $\overrightarrow{F_m}$ is given by,
\[\overrightarrow{F_e}=q\overrightarrow{E}\] \[\overrightarrow{F_m}=q(\overrightarrow{v}×\overrightarrow{B})\]
Hence, Lorentz force $\overrightarrow{F}$ on a charged particle moving with velocity $\overrightarrow{v}$ in an electric field $\overrightarrow{E}$ and magnetic field $\overrightarrow{B}$ is given by
\[\overrightarrow{F}=\overrightarrow{F_e}+\overrightarrow{F_m}\]
\[\therefore \overrightarrow{F}=q(\overrightarrow{E}+\overrightarrow{v}×\overrightarrow{B})\]
