Here, we will discuss about the different cases and analyse the motion of a body in a vertical circle.
Suppose a body of mass
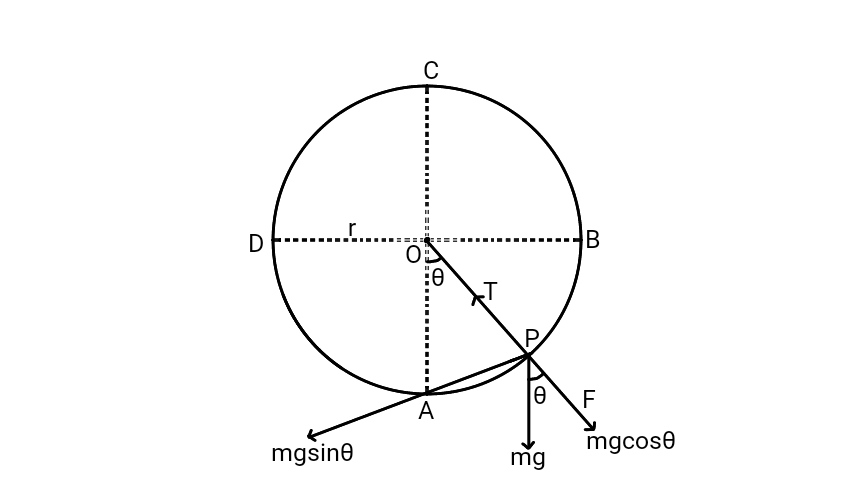
Let
Maximum Tension in the String

At the lowest point
Minimum Tension in the String

At highest point
Tension in the String at B and D
Minimum Velocity required to Loop in the Vertical Circle
At highest point
At lowest point
- If a bucket containing water is rotated along a vertical circle such that its velocity at the lowest point is equal to or greater than
- The pilot of a jet plane who is not tied to his seat will not fall down while looping the vertical circle.
- For the same reason, in circus, the motorcyclists are able to move in a vertical circle inside a cage.
More on Circular Motion
