Variation of g due to the shape of the earth
We know, $g ∝ \frac{1}{R}^2$. So, acceleration due to gravity depends upon the radius of the earth. The shape of the earth is not completely spherical. Because of this, there is difference in radius of the earth. The polar regions of the earth are little flattened whereas the earth bulges out at the equator. So, the radius of the earth at the poles is less than the radius of the earth at the equator. That’s why, the value of $g$ is maximum at the poles and minimum at the equation due to $g ∝ \frac{1}{R^2}$.
Value of $g$ at the polar region $= 9.83\text{ m/s}^2$
Value of $g$ at the equator $= 9.78\text{ m/s}^2$
For the mean value of acceleration due to gravity on the surface of the earth,
\[ \text{Mass of the earth (M) }= 6 × 10^{24} \text{ kg}\] \[ \text{Radius of the earth (R) }= 6400 \text{ km} = 6400000 \text{ m}\] \[ \text{Gravitational Constant (G) }= 6.67 × 10^{-11} \text{ Nm^2/kg^2}\] \[ \text{Acceleration due to gravity of the earth (g) }= \text{?}\]
\[\text{We know, } g = \frac{GM}{R^2}\] \[= \frac{6.67 × 10^{-11} × 6 × 10^{24}}{6400000^2}\] \[= 9.8 \text{ m/s}^2\]
Thus, the mean value of the acceleration due to gravity on the surface of the earth is $9.8\text{ m/s}^2.$
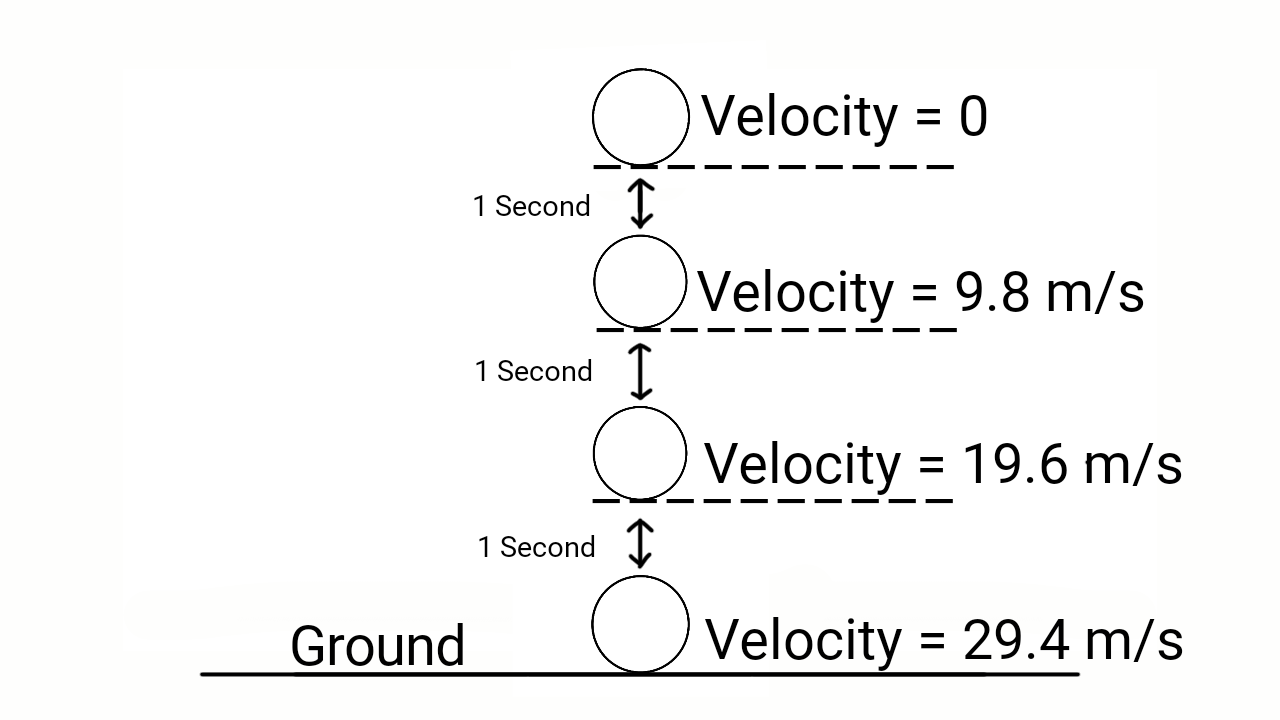
We have learnt that the acceleration due to gravity is more at the poles than at the equator. That’s why, the weight of a body is more at the poles than at the equator. And, if we dropped an object once at the equatorial regions and then at the polar regions from the same height, then that object will fall faster at the polar regions than the equatorial regions.
Variation of g due to the height from the surface of the earth
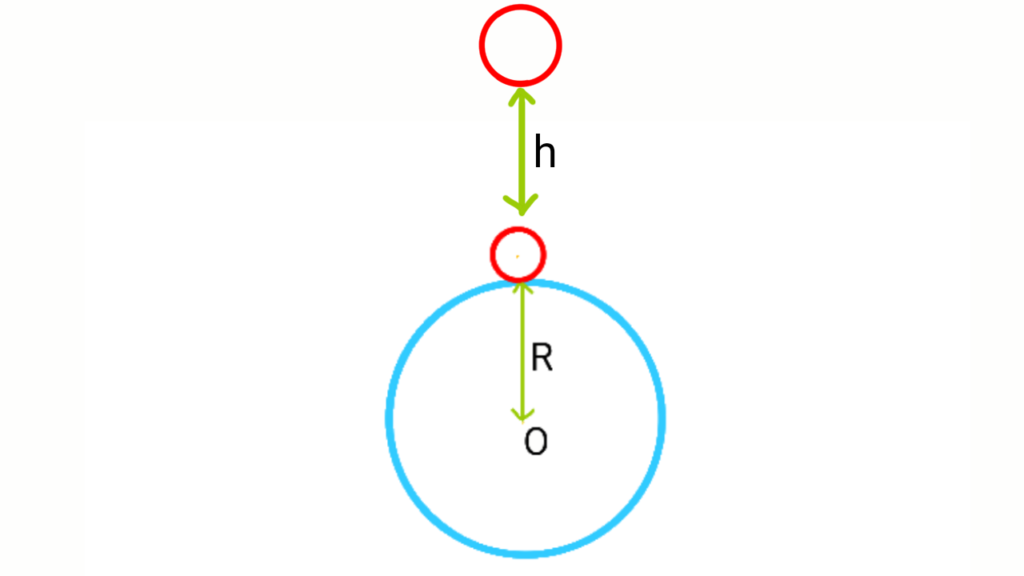
Let the mass and the radius of the earth be $M$ and $R$ respectively. Then the value of the acceleration due to gravity on the surface of the earth is,
\[g = \frac{GM}{R^2} \text{ ____(i)}\]
Let the another acceleration due to gravity at a height $h$ from the earth’s surface be $g_1$. Then,
\[g_1 = \frac{GM}{(R+h)^2} \text{ ____(ii)}\]
Dividing equation $(ii)$ by equation $(i)$,
\[\frac{g_1}{g} = \frac{\frac{GM}{(R+h)^2}}{\frac{GM}{R^2}}\] \[\frac{g_1}{g} = \frac{R^2}{(R+h)^2}\] \[g_1 = \left[\frac{R}{R+h}\right]^2g\]
Here, $\left[\frac{R}{(R+h)}\right]^2$ will be always less than $1$ in some height. Since $\left[\frac{R}{(R+h)}\right]^2$ < 1, the value of $g_1$ will be less than $g$.
Therefore, if height increases the value of the acceleration due to gravity decreases. That’s why, the weight of the body decreases as we go in higher altitude. And a body will weigh more at the sea level than at the top of the mountain.
Variation of g due to the depth from the surface of the earth
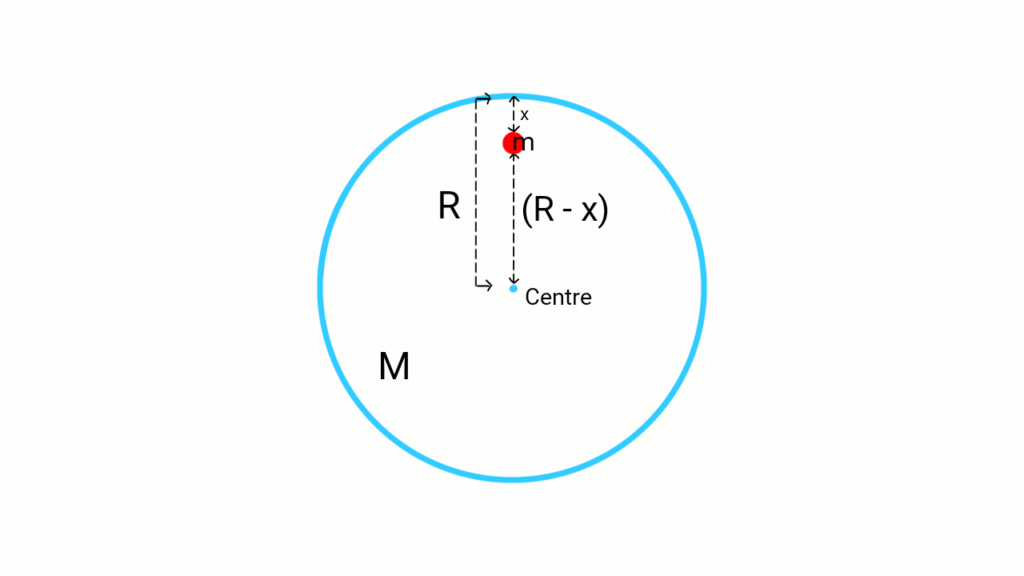
Let the mass and the radius of the earth be $M$ and $R$ respectively.
Acceleration due to gravity on the surface of the earth is,
\[g = \frac{GM}{R^2}\] \[g = \frac{Gρ \frac{4}{3}πR³}{R²}\text{ [m = density × volume]}\] \[g= Gρ \frac{4} {3} πR \text{ ____(i)}\] Where, $ρ$ is density and volume $= \frac{4}{3}πR^3$.
Let a body is at depth $x$ from the earth’s surface. Then, the acceleration due to gravity at depth $x$ is,
\[g_1 = \frac{GM}{(R-x)^2}\] \[g_1= \frac{Gρ \frac{4}{3}π(R-x)^3}{(R-x)^2}\] \[g_1= Gρ \frac{4}{3}π(R-x) \text{ ____(ii)}\]
where, $ρ$ is density and volume = $\frac{4}{3}π(R-x)^3$.
Dividing equation $(ii)$ by $(i)$,
\[\frac{g_1}{g} = \frac{Gρ\frac{4}{3}π(R-x)}{Gρ\frac{4}{3}πR}\] \[\frac{g_1}{g}= \frac{R-x}{R}\] \[g_1 = \left[\frac{R-x}{R}\right]g\] Here, The value of $\left[\frac{(R-x)}{R}\right]$ will be always less than $1$ in some depth. Thus, $g_1$ is less than $g$. So, if depth from the earth’s surface increases then the acceleration due to gravity decreases. A body will weigh less at depth than the surface. That’s, the weight of a body decreases in a coal mine.
In the centre of the earth, $x = R$. \[\text{Then, } g_1 = \left[\frac{R-R}{R}\right] g = 0\]
Thus, the acceleration due to gravity at the centre of the earth is zero and if a body is kept at the centre of the earth than its weight will be zero and it will feel weightlessness.
Variation of g due to the rotation of the earth
Let the earth be a sphere of radius $R$ and mass $M$. Place an object of mass $m$ at point $P$ at latitude $θ$. Then, the weight of the object will be $mg$ when the earth is not rotating.
But the earth is rotating. Let the earth be rotating by angular velocity $ω$. So, the object is also rotating in a circular path of radius $r$. In this case, the object experiences centrifugal force $F$. \[F=mω^2r\] \[F=mω^2R\cos θ\;\;\;[∵r=R\cos θ]\]
Two forces [$mg$ and $F$] acts on the object. Due to the centrifugal force $F$, the component of the weight of the object $PS$ [shown in figure (2)] decreases. Thus, new component of the weight along $PS$ \[=mg\cos θ-F\] \[=mg\cos θ-mω^2R\cos θ\] Due to this decrement in the component of weight, the apparent weight of the object becomes $mg’$. Now, \[\text{Resultant } PO’=\sqrt{PS^2+PN^2}\] \[mg’=\sqrt{(mg\cos θ-mω^2R\cos θ)^2+(mg\sin θ)^2}\] \[mg’=\sqrt{m^2g^2\cos^2θ-2m^2gω^2R\cos^2θ+m^2ω^4R^2\cos^2θ+m^2g^2\sin^2θ}\] \[mg’=\sqrt{m^2g^2(\cos^2θ+\sin^2θ)-2m^2gω^2R\cos^2θ+m^2ω^4R^2\cos^2θ}\] Neglecting higher power of $ω$, \[mg’=\sqrt{m^2g^2-2m^2gω^2R\cos^2θ}\] \[mg’=mg\sqrt{1-\frac{2ω^2R\cos^2θ}{g}}\] \[g’=g\left(1-\frac{2ω^2R\cos^2θ}{g}\right)^{\frac{1}{2}}\] Expanding binomially and neglecting higher power of $ω$, we get, \[g’=g\left(1-\frac{ω^2R\cos^2θ}{g}\right)\] \[g’=g-ω^2R\cos^2θ\] This gives the acceleration due to gravity due to rotation of earth. At equator, $θ=0°$, \[g’=g-ω^2R\] ∴Acceleration due to gravity is minimum at equator. At poles, $θ=90°$, \[g’=g\] ∴Acceleration due to gravity is maximum at poles.
Variation of g in different planets and satellites
As we know that the weight of an object is the force by which the object is pulled by a planet towards its centre. According to Newton’s second law of motion, \[F = ma\] \[F = mg\;\;\;(a = g)\] Thus, we can use the formula given below for the calculation of weight \[W = mg \text{ ____(i)}\] The value of $g$ is given by, \[g = \frac{GM}{R^2} \text{ ____(ii)}\] From equation $(i)$ and equation $(ii)$, we get, \[W = \frac{GMm}{R^2} \text{ ____(iii)}\] Where, $M$ and $R$ are the mass and the radius of the planet respectively. Since the value of $M$ and $R$ differs in different planet, the value of $g$ is also different in different planets. That’s why, the weight of a body is also variable in them.
As we know that the moon is smaller than the earth. So, its acceleration due to gravity is $1.67\text{ m/s}^2$ which is $(1/6)^{\text{th}}$ times of the acceleration due to gravity of the earth. Therefore, the weight of the body is $(1/6)^{\text{th}}$ times less at the moon than at the earth.
The acceleration due to gravity at Jupiter is $25\text{ m/s}^2$ which is $2.5$ times more than the acceleration due to gravity of the earth. Therefore, the weight of the body is $2.5$ times more at the Jupiter than at the earth.
