Mass
Newton’s first law of motion tells us that a force acting on a body affects its property of inertia. How much or how little the force acting on the body changes its inertia, gives rise to one of the fundamental notion of physical sciences, namely, the notion of mass of the body.
To measure or describe by how much or how little a given force acting on a body changes its amount of inertia, we may assign a numerical quantity (or factor or multiplier) that describes how much or how little it changes its state of rest or uniform motion per unit time (i.e. its acceleration) under the action of a given force. This numerical quantity is called mass of the body and is denoted by symbol $m$ (italic).
Measurement of Mass
Suppose a given force acts on two bodies of masses $m_1$ and $m_2$. Since these bodies have different masses, they will acquire different accelerations. Experiments show that a body of smaller mass acquires a greater acceleration as compared with a body of larger mass.
If $a_1$ and $a_2$ be the accelerations acquired by the masses $m_1$ and $m_2$ respectively under the action of the same force, the ratio $a_2/a_1$ may be used to compare the two masses. \[m_1=\frac{a_2}{a_1}m_2\]
The convention used it to say that the mass $m_1$ is $a_2/a_1$ times the mass $m_2$.
This equation may be used to measure the mass of a given body by comparing with a standard unit of mass. A standard unit of mass is called the kilogram $\text{(kg)}$. It is the mass of a solid cylinder made of platinum-iridium alloy kept at the International Bureau of Weights and Measures at Sivres, near Paris.
The mass of the composite body formed by fastening the bodies of masses $m_1$ and $m_2$ together can be measured and it is found to be $m_1 + m_2$. Thus, mass is found to be additive and is directly related to the quantity of matter. This explains the use of the term ‘mass‘ by Newton to mean ‘quantity of matter‘. Thus, mass of a body can also be defined as the amount of matter contained in that body.
Momentum
If a given force acts upon two bodies of different masses, then the light body acquires higher velocity than the heavier body. Also, a force applied on a greater mass is greater than a force applied on a smaller mass to acquire the same velocity after travelling the same distance from rest. Thus, we can say that the heavier body has greater quantity of motion or momentum than the lighter one.
Momentum of a body is defined as the product of its mass and its velocity. \[\text{Momentum}=\text{Mass}×\text{Velocity}\]
Its SI unit is $\text{kg m s}^{-1}$.
Momentum Change And Mass Change
If a body of mass $m$ changes its velocity from $u$ to $v$ in time $t$ under the action of a force $F$, then according to Newton’s Second Law of Motion, \[F=\frac{mv-mu}{t}\] \[F×t=mv-mu=\text{Momentum Change}\]
The quantity $F×t$ is called impulse of the force on the body.
Also, \[F=\frac{mv-mu}{t}\]
\[=\frac{m}{t}(v-u)\]
\[=\text{Mass per second}×\text{Velocity change}\]
This form of the equation of motion of the body is useful in dealing with bodies whose masses are uniformly increasing or decreasing with motion as in the cases of rainfall and rocket firing.
Q. Suppose a rocket moving upwards in the air loses its mass at the rate of $0.1$ $\text{kg s}^{-1}$ as its fuel burns. If the velocity of the rocket changes from $110$ $\text{ms}^{-1}$ to $10$ $\text{ms}^{-1}$, find the force retarding the motion of the rocket.
Let $F$ be the retarding force, $u$ be the initial velocity, $v$ be the final velocity and $r$ be the change in mass per second.
Then, \[F=\frac{m}{t}(v-u)\] \[F=r(v-u)\] \[F=0.1×(10-110)\] \[F=-10 \text{ }N\]
Q. Rain drops falling vertically on a flat roof at the rate of $0.2$ $\text{kg s}^{-1}$ come to rest after hitting the roof. If the resistance force of the roof is $2$ $N$, find the velocity of the rain.
Let $r =$ mass of water falling per sec = $0.2$ $\text{kg s}^{-1}$
$u =$ velocity before hitting roof
$v =$ velocity after hitting roof $= 0$
$F =$ resistance force $= -2 N$
Then, \[F=\frac{m}{t}(v-u)=r(v-u)\] \[-2=0.2(0-u)\] \[-2=-0.2u\] \[u=10 \text{ m/s}\]
Law of Conservation of Linear Momentum
For Single Body System
Statement:
Unless external force is applied the linear momentum of body remains conserved.
Let us consider a body having mass $m$ is moving with velocity $v$ then according to Newton’s Second Law of Motion, \[F=\frac{dp}{dt}\]
When $F=0$, \[0=\frac{dp}{dt}\] \[dp=0\]
Integrating, \[\int dp = \int 0\] \[∴ p = \text{Constant}\]
For Two Bodies System
Statement:
Unless external force is applied, the sum of linear momentum before collision is equal to the sum of linear momentum after collision.
Let us consider a body $(A)$ having mass $m_1$ is moving with velocity $u_1$ and another body $(B)$ of mass $m_2$ moving with velocity $u_2$ in a straight line in such a way that $u_1>u_2$ collide with each other and separate from each other with velocities $v_1$ and $v_2$.
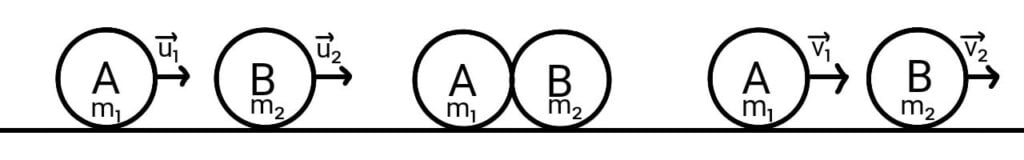
Then,
Change in momentum of body $A$ \[=m_1v_1-m_1u_1\]
Rate of change in momentum of body $A$
\[=\frac{m_1v_1- m_1u_1}{Δt}\]
\[∴F_1=\frac{m_1v_1-m_1u_1}{Δt} \text{ ____(1)}\]
Similarly,
Change in momentum of body $B$ \[=m_2v_2-m_1u_1\]
Rate of change in momentum of body $B$
\[={m_2v_2- m_2u_2}{Δt}\]
\[∴F_2 = {m_2v_2-m_2u_2}{Δt} \text{ ____(2)}\]
According to Newton’s Third Law of Motion,
\[F_1=-F_2\]
\[\frac{m_1v_1-m_1u_1}{∆t}=-\frac{m_2v_2-m_2u_2}{∆t}\]
\[m_1v_1-m_1u_1=-m_2v_2+m_2u_2\]
\[∴ m_1u_1+m_2u_2=m_1v_1+m_2v_2\]
Thus, when two bodies interact with each other and no external forces act on the system, the total momentum of the system remains constant both in magnitude and direction. This is called the Principle of Conservation of Linear Momentum.
Q. An object of mass $2$ $\text{kg}$ is moving in +ve X-direction with a velocity of $3$ $\text{ms}^{-1}$ while an object of mass $1$ $\text{kg}$ is moving in -ve X-direction with a velocity of $4$ $\text{ms}^{-1}$. They collide head on and stick together. Find their common velocity after collision.
By the law of conservation of linear momentum, \[m_1u_1+m_2u_2=(m_1+m_2)v\] \[2×3+1×(-4)=(2+1)v\] \[2=3v\] \[v=\frac{2}{3} \text{ ms}^{-1}\]
