Torque
The turning effect of force is called moment of force or torque. It is the product of magnitude of force and the perpendicular distance of the line of action of force from the axis of rotation. It is denoted by
Torque
If force
Its SI unit is
Relation Between Torque And Angular Acceleration
Consider a rigid body which has a particle of mass
Then,
This gives the torque for a single particle. But there are large number of particles in the body.
Suppose there are
Therefore, moment of inertia of a rigid body about an axis of rotation is numerically equal to the external torque required to produce a unit angular acceleration in the rotational motion of the body about that axis.
In translation motion, an external force is required to produce change in the motion of body and is given by,
In rotational motion, an external torque is required to produce change in the motion of body and is given by,
Work Done By A Torque
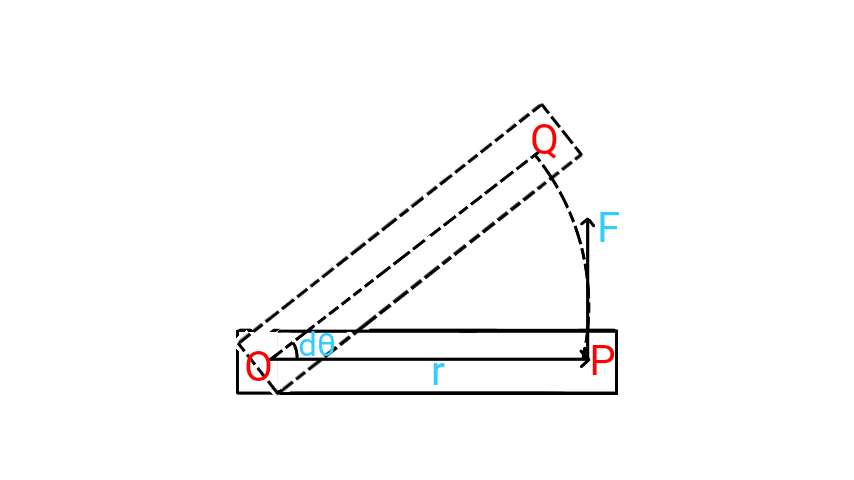
Consider a rigid body rotating about an axis passing through point
Here,
If the body is rotated through an angle
If the body moves from
Couple
Two equal and opposite parallel forces acting on a rigid body at different points constitute a couple. Such a pair of forces acting upon a rigid body cannot produce a motion of translation but produce only rotation.
The perpendicular distance between the lines of the action of the two forces is known as the arm of the couple. The moment of a couple or torque is the product of the magnitude of one of the forces forming the couple and the arm of the couple.
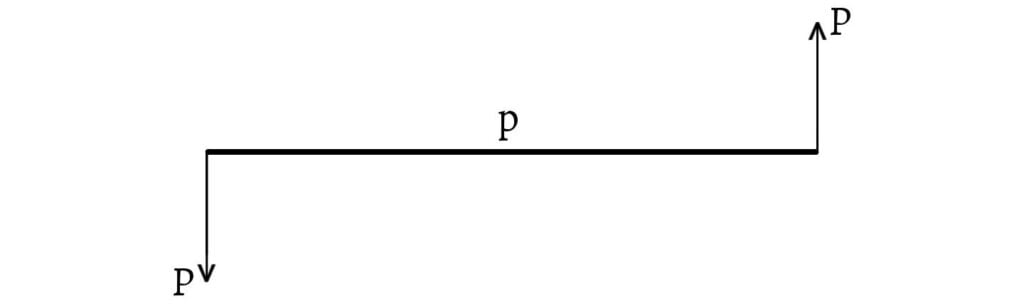
If
The forces appied to the handle of screw driver, to the lid of a bottle while opening or closing it, to the key of a clock in winding it up or to the handle of the door while opening it are some examples of couple.

Let us consider a flywheel with radius
Let
Then, the work done due to angular displacement of wheel by
Hence, work done by couple is the product of torque applied and angular displacement of rotational body.
Theorems of Couple
Theorem 1: The algebraic sum of the moments of two forces forming a couple about any point in their plane is constant and equal to the moment of the couple.
Let
Case I:
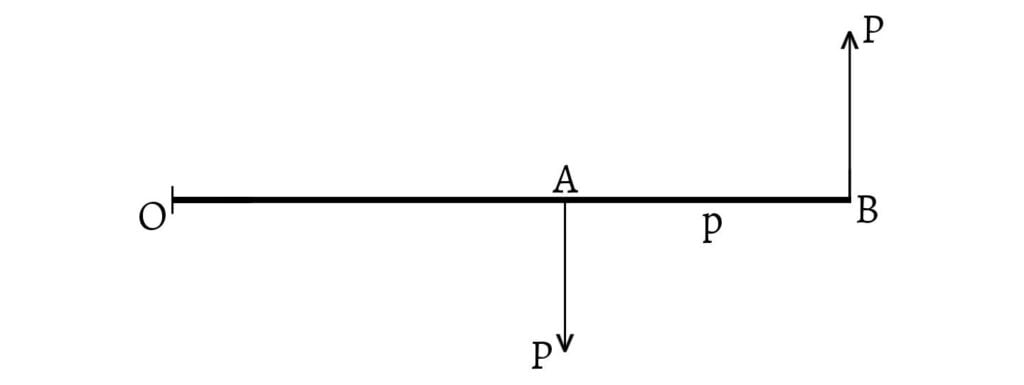
The algebraic sum of the moments of the forces about
Case II:
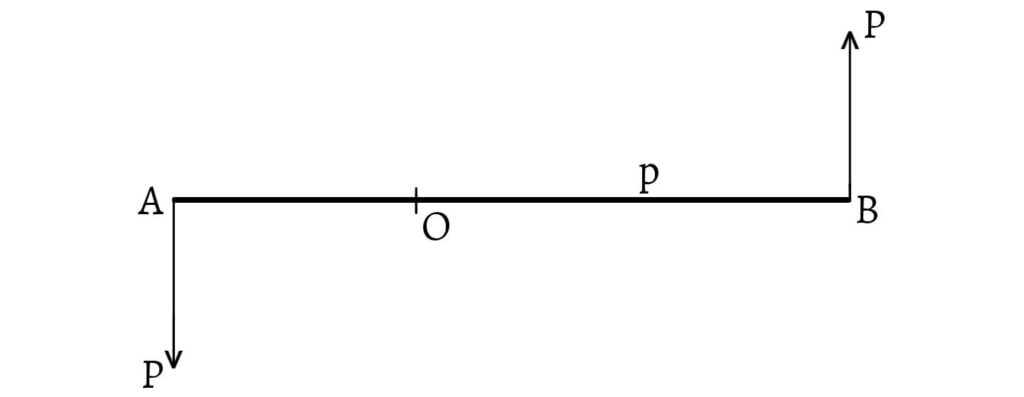
The algebraic sum of the moments of the forces about
Case III:

The algebraic sum of the moments of the forces about
Hence, in every cases, the algebraic sum of the moments is constant and equal to the moment of the couple wherever maybe the point
Theorem 2: Two couples, acting in the same plane upon a rigid body, whose moments are equal in magnitude but opposite in sign, balance one another.
Let
Case I: When the forces forming the couples are not parallel

Let one of the forces
Draw
The two forces
The sum of the moments of
Thus, the resultant of the forces forming the two couples vanish and hence the couples balance one another.
Case II: When the forces forming the couples are parallel

Draw a straight line perpendicular to the lines of action of the forces, meeting them at
Since the moments of the couples are equal in magnitude,
Let the resultant
Subtracting
Thus, the resultant
Cor. Two couples in the same plane, having equal moments are equivalent to one another.
Theorem 3: The effect of a couple upon a rigid body is unaltered if it is transferred to any plane parallel to its own.
Let
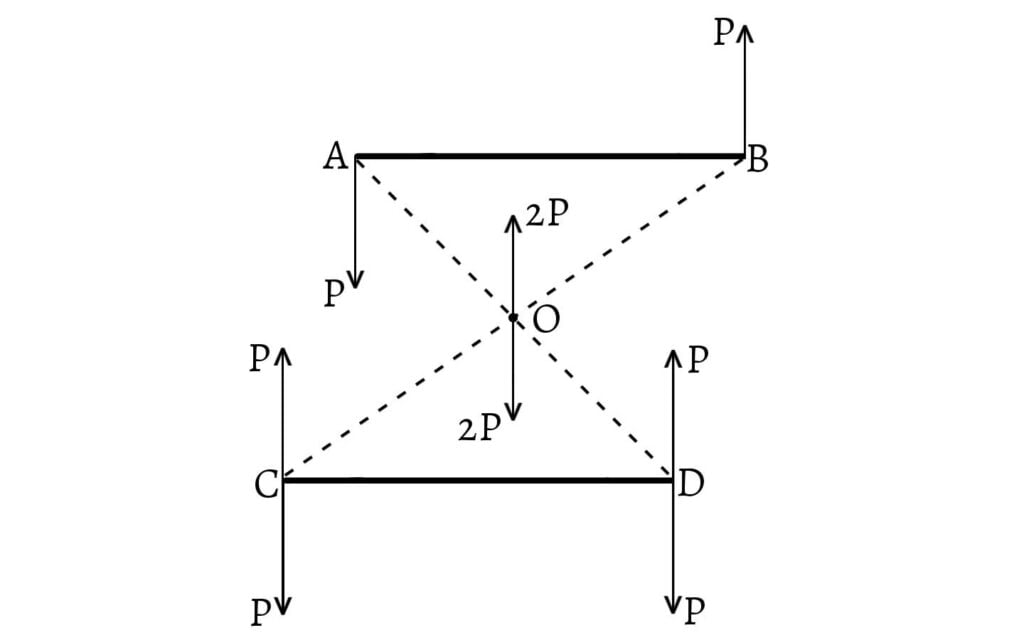
At
Cor. A couple may be replaced by any other couple acting in a parallel plane, provided the moments of the two couples are the same.
Theorem 4: Any number of couples in the same plane acting on a rigid body are equivalent to a single couple whose moment is equal to the algebraic sum of the moments of the couples.
Let

Since the couples of equal moments in the sme plane are equivalent, the couple
Hence, by the couple
Similarly, the couple
If we replace all the couples in this way, then a single couple is obtained with the arm
Hence, the moment of the single couple is equal to
Theorem 5: A force and a couple acting in the same plane upon a rigid body are equivalent to a single force, equal and parallel to the original single force.
Let

Replace the given couple by another couple having its forces each equal to
Now, place the couple such that one of its component force

The two equal forces acting along
Theorem 6: If three forces, acting upon a rigid body, be represented in magnitude, direction and line of action by the sides of a triangle taken in order, they are equivalent to a couple whose moment is represented by twice the area of the triangle.
Let

Draw
Hence, two forces are left;
Hence, three forces represented by the sides of a traingle taken in order are equivalent to a couple whose moment is equal to the twice the area of the triangle.
