Brewster’s law is a relation between refractive index of a given medium and polarizing angle for the medium. This law is used to produce polarized light by reflection.
In 1808, Malus discovered that when ordinary light is reflected from a surface of a transparent medium like glass or water, it becomes partly polarized. The degree of polarization varies with the angle of incidence.
In 1812, Brewster performed various experiments to study the polarization of light by reflection at different surfaces. He observed that, for a particular angle of incidence, the reflected light is completely polarized in the plane of incidence. This particular angle of incidence is called Brewster’s angle or polarizing angle or angle of polarization. Its value depends upon the nature of the medium on which the light is incident and wavelength of the light used.
The angle of incidence at which the reflected light is completely polarized is called polarizing angle.
Brewster’s law states that
“The refractive index of a medium is equal to the tangent of the polarizing angle for the given medium.”
Mathematically, \[μ=\tan Φ\] Where, $μ$ is the refractive index of the given medium and $Φ$ is the polarizing angle.
Derivation of Brewster’s Law
Let $AB$ be an incident unpolarized light striking a medium of refracting index $μ$ on the surface $PQ$ at polarizing angle $Φ$ which undergoes refraction along $BD$. Then, the reflected light $BC$ is completely polarized.
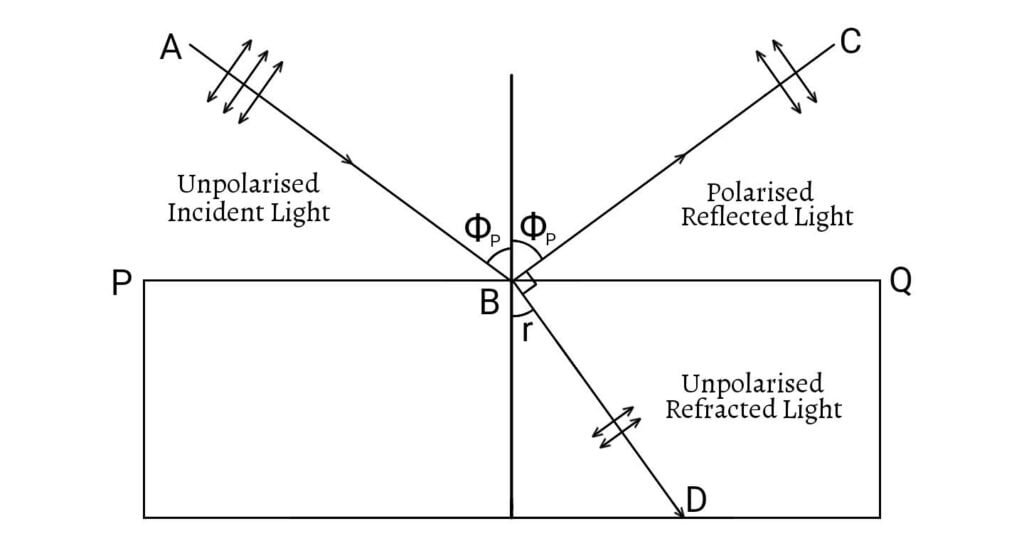
From Snell’s law, we have, \[μ=\frac{\sin i}{\sin r}=\frac{\sin Φ}{\sin r} \text{ ____(1)}\]
From his experiments, Brewster also found that the reflected ray and the refracted ray are perpendicular to each other for a polarizing angle.
\[\therefore Φ+r=90\] \[r=90-Φ\text{ ____(2)}\]
From $(1)$ and $(2)$, \[μ=\frac{\sin Φ}{\sin(90-Φ)}=\frac{\sin Φ}{\cos Φ}\] \[μ=\tan Φ\] This is Brewster’s law.
[Also See: Wave Theory of Light]
