Ampere’s circuital law is analogous to Gauss’s law in electrostatics. In Gauss’s law, net charge is taken in a closed surface to solve for electric field. In Ampere’s law, net current is taken in a closed surface to find the magnetic field. In order to illustrate the use of Ampere’s law, we consider the following cases:
- Magnetic field at a point due to a current carrying long straight conductor
- Magnetic field at a point on the axis of a solenoid carrying current
Magnetic field at a point due to a current carrying long straight conductor
Consider a long straight conductor carrying current $I$ in the upward direction. A point $P$ at a perpendicular distance $r$ from the conductor is taken where magnetic field is to be calculated.

If we choose a circle of radius $r$ as the Amperian loop, the magnitude of $\overrightarrow{B}$ is same at every point on the path and the angle between $\overrightarrow{B}$ and $\overrightarrow{dl}$ is zero at every point on the circle. Thus, applying Ampere’s law to this closed path, we get, \[\oint \overrightarrow{B}.\overrightarrow{dl}=\mu_oI\] \[\oint B.dl\cos0°=\mu_oI\] \[\oint B.dl=\mu_oI\] \[B\oint dl=\mu_oI\] \[B×2πr=\mu_oI\] \[\therefore B=\frac{\mu_oI}{2πr}\] This gives the magnetic field at a point due to a current carrying long straight conductor.
Magnetic field at a point on the axis of a solenoid carrying current
Consider a long solenoid having $n$ number of turns per unit length and carrying current $I$. Inside the solenoid, the magnetic field $(\overrightarrow{B})$ is uniform, strong and directed along the axis of the solenoid. The magnetic field outside the solenoid is very weak and can be neglected. In order to use Ampere’s law, a rectangular Amperian loop $PQRSP$ is considered where $PQ=l$.
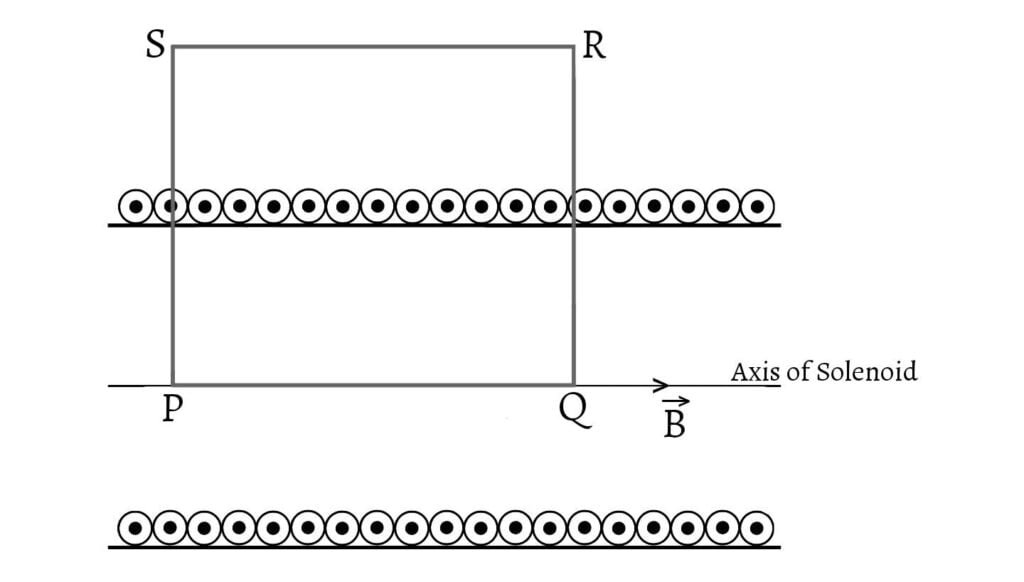
The line integral of $\overrightarrow{B}$ over the closed path $\text{PQRSP}$ is given by,
\[\oint \overrightarrow{B}. \overrightarrow{dl}\] \[=\int_P^Q \overrightarrow{B}. \overrightarrow{dl}+\int_Q^R \overrightarrow{B}. \overrightarrow{dl}+\int_R^S \overrightarrow{B}. \overrightarrow{dl}+\int_S^P \overrightarrow{B}. \overrightarrow{dl}\text{ __(1)}\]
Here, \[\int_P^Q \overrightarrow{B}. \overrightarrow{dl}=\int_P^Q B.dl\cos0°\] [Angle between $\overrightarrow{B}$ and $\overrightarrow{dl}$ is $0°$]
\[\therefore \int_P^Q \overrightarrow{B}. \overrightarrow{dl}=Bl\] \[\int_Q^R \overrightarrow{B}. \overrightarrow{dl}=\int_Q^R B.dl\cos90°\] [Angle between $\overrightarrow{B}$ and $\overrightarrow{dl}$ is $90°$]
\[\therefore \int_Q^R \overrightarrow{B}. \overrightarrow{dl}=0\] \[\int_R^S \overrightarrow{B}. \overrightarrow{dl}=0\] [Field outside the solenoid is neglected]
\[\int_S^P \overrightarrow{B}. \overrightarrow{dl}=\int_S^P B.dl\cos90°=0\]
Putting these values in equation $(1)$, we get,
\[\oint \overrightarrow{B}. \overrightarrow{dl}=Bl+0+0+0\] \[\oint \overrightarrow{B}. \overrightarrow{dl}=Bl \text{ __(2)}\]
From Ampere’s law, we have, \[\oint \overrightarrow{B}. \overrightarrow{dl}=\mu_o × \text{ net current}\text{ __(3)}\]
Here, $\text{number of turns for unit length} = n$ $\text{number of turns for length } l=nl$ $\text{current in each turn}=I$ $\therefore \text{net current through PQRS}=nl×I$
From $(3)$, \[\oint \overrightarrow{B}. \overrightarrow{dl}=\mu_o×nIl\text{ __(4)}\]
From $(2)$ and $(4)$, \[Bl=\mu_onIl\] \[\therefore B=\mu_onI\]
This gives the magnetic field within an infinitely long solenoid.
[Also See: Determination of Magnetic Field at a Point on the Axis of a Solenoid by using Biot and Savart Law]
