Slope Intercept Form

Here, we will discuss about the slope intercept form of the equation of straight lines.

Let a straight line intersect x-axis and y-axis at $A$ and $B$ respectively. Let $AB$ make an angle $\theta$ with the positive x-axis. Then \[\text{ Slope of the line }(m)=\tan\theta\]

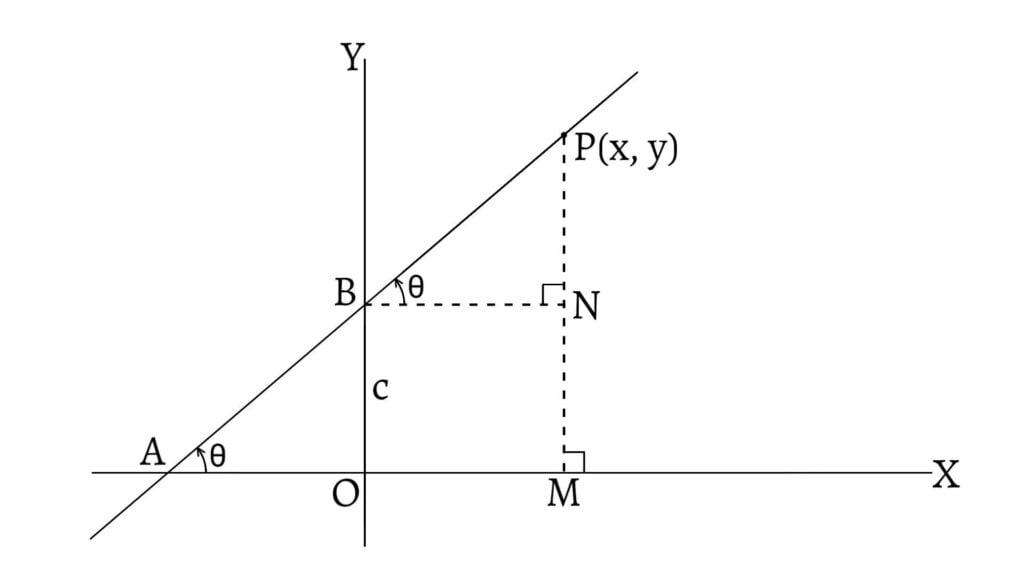
Let $OB=c$ and $P(x,y)$ be any point on the line. Draw $PM$ perpendicular to x-axis and $BN$ parallel to x-axis. Then, \[\tan\theta=\frac{NP}{BN}\] \[m=\frac{PM-MN}{OM}\] \[m=\frac{y-c}{x}\] \[mx=y-c\] \[\therefore y=mx+c\]

Since this equation is satisfied by the coordinates $(x,y)$ of any point $P$ on the line, it is the equation of the line. This equation is referred to as the slope intercept form or simply the slope form of an equation of a straight line.

• If the line passes through the origin, $c=0$, and the equation will be $y=mx$.
• If the line be parallel to the x-axis (slope $m=0$), the equation becomes $y=c$.
• If the line be parallel to the y-axis, then the equation cannot be written in this form.

Find the equation of a line making an angle $60°$ with the positive x-axis and cutting an intercept $3$ from the y-axis.

Here, \[\text{y-intercept } (c)=3\] \[\theta=60°\] \[\therefore \text{Slope }(m)=\tan 60°=\sqrt{3}\]

Thus, equation of straight line is \[y=mx+c\] \[\therefore y=\sqrt{3}\text{ }x+3\]

---

Find the equation of a straight line making an angle of $135°$ with the positive x-axis and cutting an intercept $3$ from the negative y-axis.

Here, \[\text{y-intercept }(c)=-3\] \[\theta=135°\] \[\therefore\text{Slope }(m)=\tan 135°=-1\]

Thus, equation of straight line is \[y=mx+c\] \[y=-x-3\] \[\therefore y+x+3=0\]

---

Find the equation of the straight lines bisecting the angle between the axes.

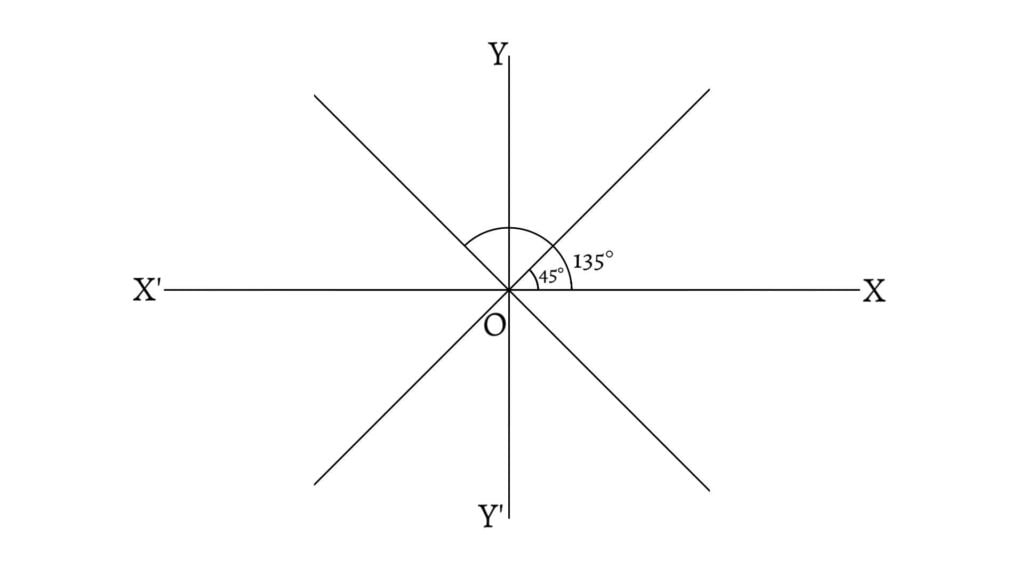
Since the line bisects the angle between the axis, $\theta=45°\text{ or }135°$. \[\therefore\text{Slope }(m)=\tan 45°\text{ or }\tan 135°\] \[=1\text{ or }-1\] \[=\pm 1\]

Thus, equation of the line is \[y=mx\] \[\therefore y=\pm x\]

---

---