Gravitational Field
Gravitational force is an action at a distance force i.e. this force exists between two masses even when they are not in contact with each other. Gravitational field is the space surrounding a material body in which its gravitational force of attraction can be measured.
Gravitational Field Intensity
Gravitational field intensity due to a material body at a point in the field is defined as the force experienced by unit mass placed at a point, provided that unit mass itself does not produce any change in the field of the material body.
Consider a material body of mass $M$ located at a point $O$. Suppose a body of mass $m$ is placed at a point $A$ which is $r$ distance apart from the centre of the material body. We have to determine the gravitational field intensity at the point $A$.

Now, Gravitational force on mass $m$ due to the material body is, \[F=\frac{GMm}{r^2}\] If $E$ is the gravitational field intensity then, \[E=\frac{F}{m}\] \[∴E=\frac{GM}{r^2}\]
This gives the gravitational filed intensity at a point which is at distance $r$.
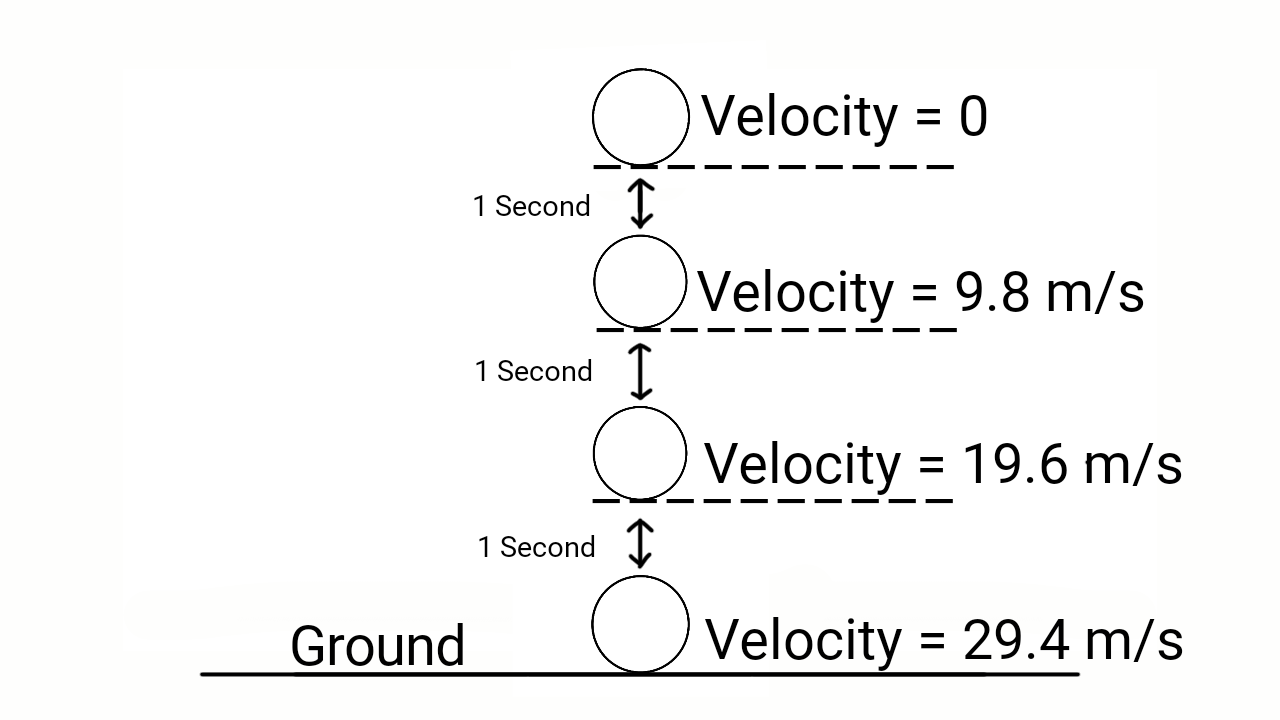
If the material body of mass $M$ is earth and the body of mass $m$ lies very close to the surface of the earth, then, $r = R$ (Radius of the earth) and, \[E=\frac{GM}{R^2}\] also, we have, \[g=\frac{GM}{R^2}\] Therefore, \[E=g\]
Thus, the gravitational field intensity near to the surface of the earth is approximately equal to its acceleration due to gravity.
Gravitational Potential Energy
Gravitational Potential Energy of a planet or body at a point is the amount of work done in bringing the body from infinity to that point inside the gravitational field of that planet.
Consider earth to be a uniform sphere of radius $R$ and mass $M$. Suppose a body is placed at a point $A$ which is $r$ distance apart from the centre of the earth. Then, we have to determine the gravitational potential energy of the body.

By definition, gravitational potential energy of the body at the point $A$ is,
$W =$ Work done in bringing the body from $∞$ to $A$.
Suppose the body is at a point $P$ which is $x$ distance apart from the centre of the earth. Then, the gravitational force of the earth on the body is given by, \[F=\frac{GMm}{x^2}\]
Small work done to move the body through infinitesimally small distance $dx$ is given by, \[dW=F\;dx\] \[dW={GMm}{x^2}\;dx\]
Integrating within limits from $∞$ to $r$, \[\int_0^W\;dW=\int_∞^r \frac{GMm}{x^2}\;dx\] \[W=GMm\int_∞^r x^{-2}\;dx\] \[W=GMm\left[ \frac{x⁻¹}{-1} \right]_∞^r\] \[W=-GMm\left[ \frac{1}{r}-\frac{1}{∞} \right]\] \[W=-\frac{GMm}{r}\]
It gives the potential energy of body of mass $m$ which is located at a distance $r$ from the centre of the earth. It is denoted by $U$.
\[∴U=-\frac{GMm}{r}\] The negative sign shows that the work is done to bring the body from infinity to a distance $r$.
Gravitational Potential
Gravitational Potential of a planet or body at a point is the amount of work done in bringing a body of unit mass from infinity to the point. It is denoted by $V$. We can obtain gravitational potential at a distance $r$ from earth’s centre by, \[V=\frac{U}{m}\] \[V=-\frac{GM}{R}\]