In nature, two or more forces are found to act simultaneously at a single point. But the effect of a single force is observed. That single force is the resultant of all the other forces and the other forces are its components. The process of finding the resultant force is known as composition of forces.
A single force whose effect is same as the sum total of the effects of a number of forces acting at a point can be found in the following ways:
Resultant of concurrent forces acting along the same line
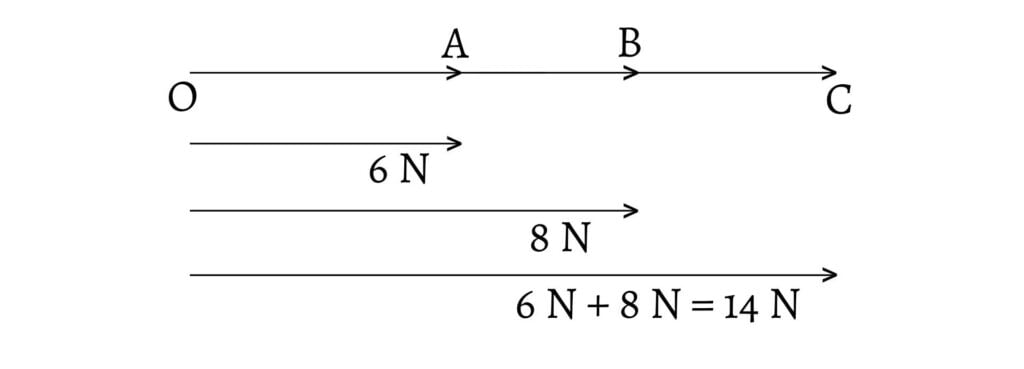
If two forces $P$ and $Q$ act on a particle in the same direction, then their resultant is the sum $P+Q$ of the forces, and acts along their common direction of action. For example, if two forces of magnitude $6N$ and $8N$ acting on a particle at $O$ are represented vectorically by $\overrightarrow{OA}$ and $\overrightarrow{OB}$ both in magnitude and in eastward direction and their resultant is $\overrightarrow{OC}$, then,
\[\overrightarrow{OC}=\overrightarrow{OA}+\overrightarrow{OB}\]\[=6N+8N=14N\]
If two collinear forces $P$ and $Q$ act on a particle but in opposite directions, their resultant is the difference $P\sim Q$ os the forces, and the resultant acts in the direction of the greater force. For example, if two forces of magnitude $6N$ and $8N$ ac the acting on a particle at $O$ are represented vectorically by $\overrightarrow{OA}$ and $\overrightarrow{OB}$ both in magnitude and in direction the first one eastward and the second one westward, and their resultant is $\overrightarrow{OC}$, then,
\[\overrightarrow{OC}=\overrightarrow{OB}-\overrightarrow{OA}\] \[=8N-6N=2N\] acting along the direction of $\overrightarrow{OB}$ i.e. westward.

Resultant of two forces acting on a particle but inclined to each other
There are two laws of vector addition that can be used to find the resultant of such forces.
Triangle Law of Vector Addition
If two forces in magnitude and direction starting from a point represents two sides of a triangle in same order, then the third side of the triangle taken in reverse order represents the resultant magnitude and direction of those two forces.
See more on Triangle Law of Vector Addition (Laws of Vector Addition)
Parallelogram Law of Vector Addition
If two forces starting from a point represents two adjacent sides of a parallelogram, then the diagonal starting from the same point represents the resultant magnitude and direction of those two forces.
See more on Parallelogram Law of Vector Addition (Laws of Vector Addition)
Alternative Method
Let $\overrightarrow{P}$ and $\overrightarrow{Q}$ be two forces acting at $O$ and $\theta$ be the angle between them.
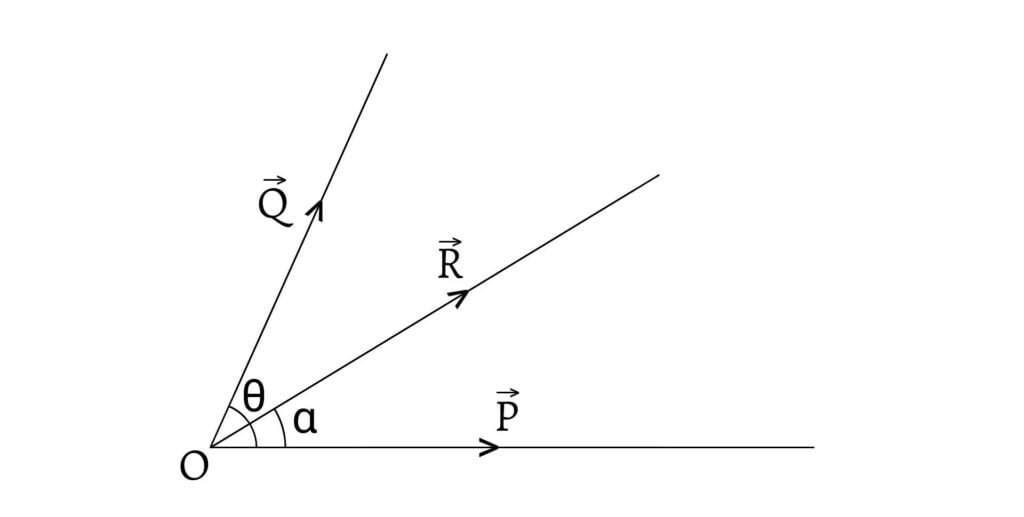
If $\overrightarrow{R}$ is the resultant of $\overrightarrow{P}$ and $\overrightarrow{Q}$, then, \[\overrightarrow{R}=\overrightarrow{P}+\overrightarrow{Q}\] \[\therefore R^2=\overrightarrow{R}.\overrightarrow{R}\] \[=(\overrightarrow{P}+\overrightarrow{Q})(\overrightarrow{P}+\overrightarrow{Q})\] \[=P^2+Q^2+2\overrightarrow{P}.\overrightarrow{Q}\] \[=P^2+Q^2+2PQ\cos\theta\] \[\therefore R=\sqrt{P^2+Q^2+2PQ\cos\theta}\] which gives the magnitude of the resultant $\overrightarrow{R}$.
For the direction of resultant $\overrightarrow{R}$, \[\overrightarrow{R}=\overrightarrow{P}+\overrightarrow{Q}\] \[\overrightarrow{P}.\overrightarrow{R}=\overrightarrow{P}.\overrightarrow{P}+\overrightarrow{P}.\overrightarrow{Q}\] \[PR\cos\alpha=P^2+PQ\cos\theta\]
where, $\alpha$ is the angle between $\overrightarrow{P}$ and $\overrightarrow{R}$. \[\therefore R\cos\alpha=P+Q\cos\theta\text{ __(1)}\]
Again, \[\overrightarrow{R}=\overrightarrow{P}+\overrightarrow{Q}\] \[\overrightarrow{P}×\overrightarrow{R}=\overrightarrow{P}×\overrightarrow{P}+\overrightarrow{P}×\overrightarrow{Q}\] \[\overrightarrow{P}×\overrightarrow{R}=\overrightarrow{P}×\overrightarrow{Q}\] \[|\overrightarrow{P}×\overrightarrow{R}|=|\overrightarrow{P}×\overrightarrow{Q}|\] \[PR\sin\alpha=PQ\cos\theta\] \[R\sin\alpha=Q\cos\theta\text{ __(2)}\]
Dividing $\text{(2)}$ by $\text{(1)}$, \[\tan\alpha=\frac{Q\sin\theta}{P+Q\cos\theta}\] \[\alpha=\tan^{-1}\left(\frac{Q\sin\theta}{P+Q\cos\theta}\right)\] which gives the direction of resultant $\overrightarrow{R}$.
Above mentioned methods and laws of vector addition provide a same result. Let $P$ and $Q$ be the two forces acting at a point by making an angle $\theta$ between them. Then the resultant $R$ of the two forces is given by, \[R=\sqrt{P^2+Q^2+2PQ\cos\theta}\]
Let $\alpha$ be the angle made by the resultant $R$ with $P$, then, \[\tan\alpha=\tan^{-1}\left(\frac{Q\sin\theta}{P+Q\cos\theta}\right)\]
For example, let two forces of magnitude $6N$ and $8N$ acting on a particle at $O$ are represented by $\overrightarrow{OA}$ and $\overrightarrow{OB}$ both in magnitude and direction the first one eastward and the second one northward and their resultant is $\overrightarrow{OC}$.

\[\overrightarrow{OC}=\sqrt{OA^2+OB^2+2.OA.OB.\cos90°}\] \[=\sqrt{6^2+8^2}=10N\] at an angle, \[\alpha=\tan^{-1}\left(\frac{8×\sin90°}{6+8\cos90°}\right)\] \[=\tan^{-1}\left(\frac{8}{6}\right)\] \[=\tan^{-1}\left(\frac{4}{3}\right)\text{ with }\overrightarrow{OA}\]
Resultant of any number of coplanar forces acting at a point
Let $P_1,P_2,P_3,…$ be the coplanar forces acting at a point $O$ and are inclined at angles $\alpha_1,\alpha_2,\alpha_3,…$ respectively to a line $OX$ through $O$. $OX$ and $OY$ are perpendicular to each other.

Then, the forces can be resolved along $OX$ and $OY$. The force $P_1$ may be replaced by its resolved parts $P_1\cos\alpha_1$ along $OX$ and $P_1\sin\alpha_1$ along $OY$. Similarly, $P_2$ may be replaced by its resolved parts $P_2\cos\alpha_2$ along $OX$ and $P_2\sin\alpha_2$ along $OY$. All the other forces are replaced in the same way. Then, Let $R$ be the resultant of the forces and $\theta$ be the angle it makes with $OX$. Then, $R$ can be resolved into $R\cos\theta$ along $OX$ and $R\sin\theta$ along $OY$.
Since the resolved parts of the resultant are equal to the algebraic sum of the resolved parts of the forces along the same direction, we get,
\[R\cos\theta=P_1\cos\alpha_1+P_2\cos\alpha_2+P_3\cos\alpha_3+…\] \[=X\text{ (let)}\] \[R\sin\theta=P_1\sin\alpha_1+P_2\sin\alpha_2+P_3\sin\alpha_3+…\] \[=Y\text{ (let)}\]
Squaring and adding, \[R^2=X^2+Y^2\] \[R=\sqrt{X^2+Y^2}\] and, by dividing, \[\tan\theta=\frac{Y}{X}\] \[\theta=\tan^{-1}\left(\frac{Y}{X}\right)\]
These equations give the magnitude of the resultant $\text{(R)}$ and its direction $(\theta)$.
[Also See: Resolution of Forces]
Forces $1N$, $2N$ and $3N$ act at a point in direction parallel to the side of an equilateral triangle taken in order. Find their resultant.
Let $ABC$ be the equilateral triangle and let the forces $1N$, $2N$ and $3N$ act at $O$ parallel to the sides $AB$, $BC$ and $CA$ of equilateral triangle $ABC$ respectively.

Resolving the forces along and perpendicular to $BC$,
\[X=2\cos0°+3\cos120°+1\cos240°\] \[=2-\frac{3}{2}-\frac{1}{2}=0\] \[Y=2\sin0°+3\sin120°+1\sin240°\] \[=0+3×\frac{\sqrt{3}}{2}-\frac{\sqrt{3}}{2}=\sqrt{3}\] \[\therefore \text{Resultant force }(R)=\sqrt{X^2+Y^2}\] \[=\sqrt{0+3}=\sqrt{3}N\]
Let $\theta$ be the angle made by the resultant with $2N$ force. \[\theta=\tan^{-1}\left(\frac{Y}{X}\right)\] \[\theta=\tan^{-1}(\infty)\] \[\therefore \theta=90°\]
Thus, resultant force is $\sqrt{3}$N and it acts perpendicular to the $2N$ force.
