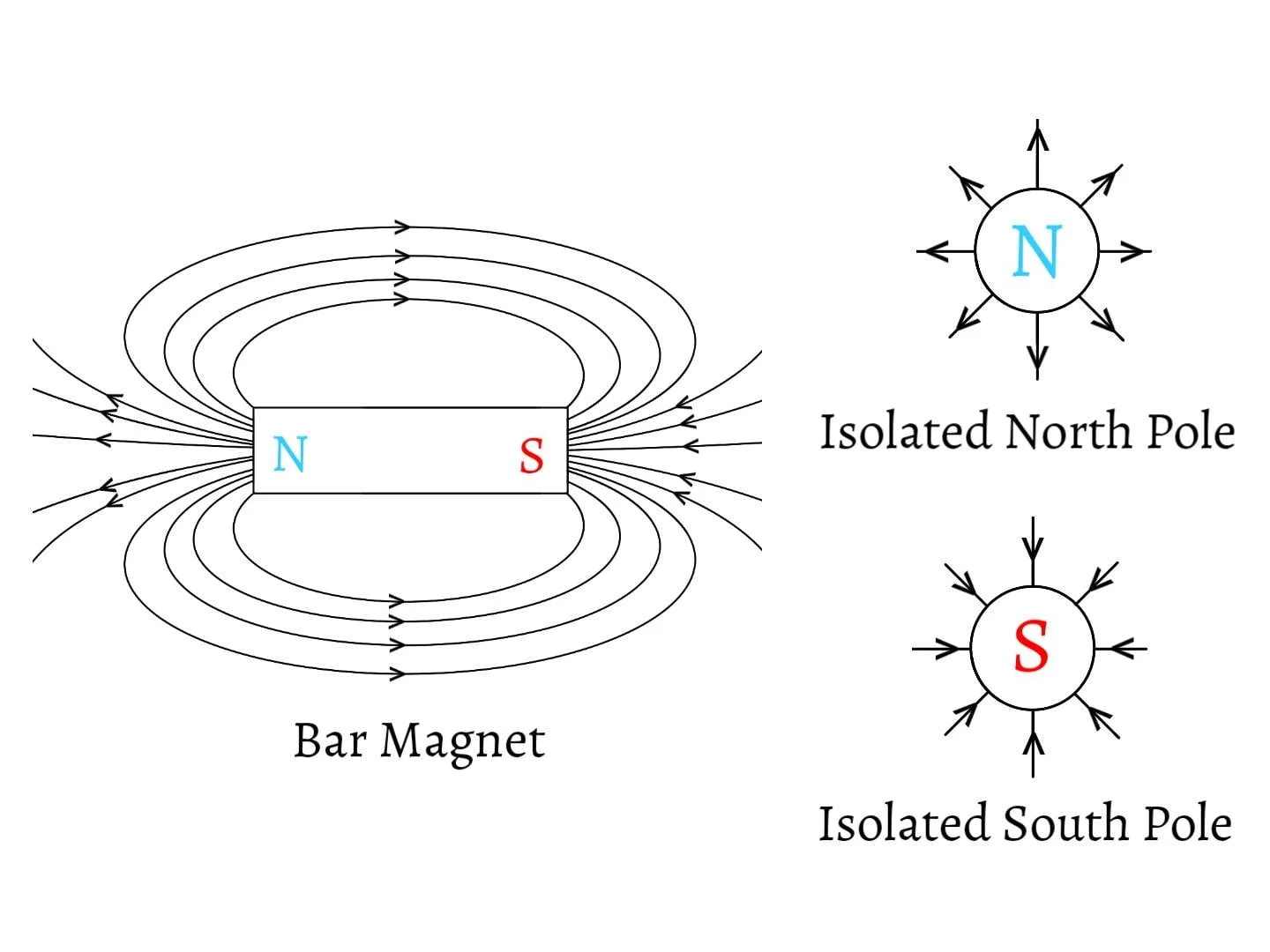
Magnetic flux through any surface area is defined as the total number of magnetic lines of force passing through that surface.
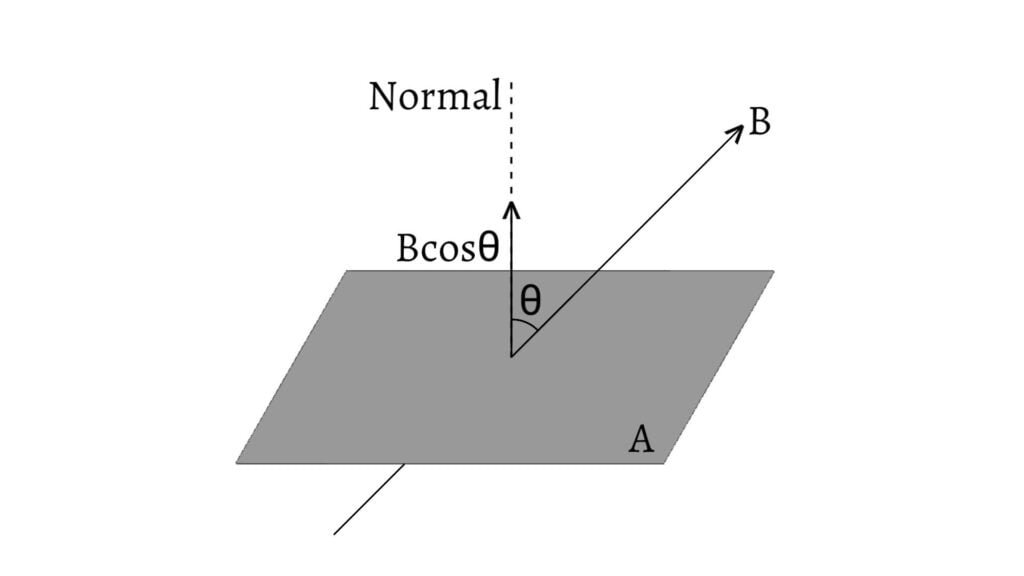
Let us consider a uniform magnetic field of strength $B$ acting on a small surface area $A$ making an angle $\theta$ with the normal on the surface. Then, magnetic flux $(\phi)$ is measured by the dot product of magnetic field $(\overrightarrow{B})$ and area vector $(\overrightarrow{A})$.
\[\therefore\phi=\overrightarrow{B}\cdot\overrightarrow{A}\]
\[\phi=BA\cos\theta\]
Magnetic flux $(\phi)$ is a scalar quantity because it is a scalar product of vector $(\overrightarrow{B})$ and area vector $(\overrightarrow{A})$.
- If magnetic field $(B)$ is parallel to the surface (i.e. angle between $B$ and the normal $(\theta)$ is $90°$) then \[\phi=BA\cos 90°=0\text{ [minimum value]}\]
- If magnetic field $(B)$ is perpendicular to the surface (i.e. angle between $B$ and the normal $(\theta)$ is $0°$) then \[\phi=BA\cos 0°=BA\text{ [maximum value]}\]
In SI unit, magnetic flux is measured in Weber $(\text{Wb})$.
If $B=1\text{ T}$, $A=1\text{ m}^2$ and $\cos\theta=1$, then we have
\[\phi=BA\cos\theta=1\text{ Wb}\]
Hence, magnetic flux is said to be of $1\text{ Wb}$ if a uniform magnetic field of $1\text{ T}$ acts normal to the area of $1\text{ m}^2$.
Magnetic Flux Density
The number of magnetic lines of force per unit normal area is called magnetic flux density. It is also known as magnetic field strength or magnetic field induction.
Mathematically, \[\text{Magnetic Flux Density}=\frac{\text{Magnetic Flux}}{\text{Normal Area}}\]
\[B=\frac{\phi}{A}\]
Its SI unit is $\text{Wb m}^{-2}$ which is called Tesla $\text{T}$. \[\therefore 1\text{ T}=1\text{ Wb m}^{-2}\]
Another non SI unit commonly in use is Gauss $\text{(G)}$ which is related to Tesla as $1\text{ T}=10^4\text{ G}$.
[Learn more about Magnetic Flux Density: Magnetic Flux Density (Magnetic Vectors)]
Flux Linkage
Consider a coil of $N$ number of turns having cross sectional area of each turn $=A$. Let the coil be placed in a magnetic field of strength $B$ which makes an angle $\theta$ with the normal to the plane of the coil. Then, the total magnetic flux passing through the coil is called flux linkage.
\[\therefore\text{Flux Linkage }(\phi)=NBA\cos\theta\]