There are two ways of transfer of energy;
1. By the transfer of matter.
2. Without the transfer of matter.
Second method is a wave process. In this process, disturbance carries energy from one place to another without the transfer of matter. The disturbance is called a wave and the process is called wave motion.
Thus, a wave is defined as a disturbance which propagates from one place to another without net transport of matter and the process of transfer of the disturbance is called wave motion. Sound, light, water ripples, etc. are the examples of wave.
Characteristics of Wave Motion
- Wave motion is a disturbance propagating in a medium.
- It transfers energy as well as momentum from one point to another.
- When it propagates in a medium, medium particles execute vibration about their mean position i.e. their motion is SHM.
- Wave motion has finite and fixed speed given by \[v=fλ\] Where, $v$ is velocity, $f$ is frequency and $λ$ is wavelength of the wave.
- The wave speed is different from particle velocity in a medium.
- When a wave travels in a medium, there is a continuous phase difference among the successive medium particles.
- The vibrating particles of the medium possess both kinetic energy and potential energy.
- It follows reflection, refraction, interference and diffraction but polarization is only for transverse wave.
Types of Wave
There are three types of wave motion;
1. Electromagnetic wave or non-mechanical wave
The wave which does not require a medium for its propagation is known as electromagnetic wave. Light, heat, radio waves etc. are electromagnetic waves.
2. Mechanical wave
The wave which requires a medium for its propagation is known as mechanical wave. Sound wave, water waves, waves on pipes and strings, seismic waves, etc. are mechanical waves.
3. Matter wave
The wave associated with a moving matter is known as matter wave. It is explained on the basis of quantum mechanics.
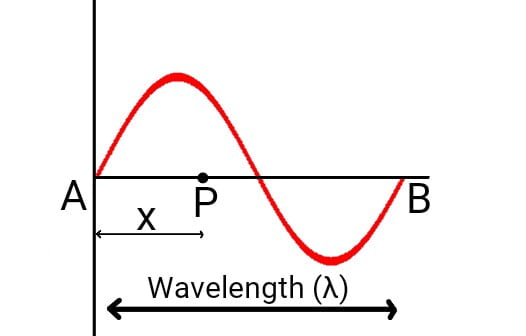
Wavelength (λ)
The distance travelled by a wave in one time period is called wavelength $(λ)$. It is the distance between any two nearest points which are in the same phase.
Frequency (f)
The number of oscillations/vibrations/cycles per second is called frequency $(f)$. It may also be defined as the number of waves passing through a point per unit time. \[f=\frac{\text{Number of cycles}}{\text{time}}\] Its unit is $\text{Hertz}$ $\text{(Hz)}$. $1\;\text{Hz} = 1 \;\text{cycle/second}$.
When a sinusoidal wave passes through a medium, every particle in the medium undergoes SHM with the same frequency. The frequency of the wave is the frequency of the vibrating source.
Time Period (T)
The time for one complete cycle is called time period $(T)$. \[T=\frac{1}{f}\]
Wave Speed
The linear distance covered per unit time by the wave is called wave speed $(v)$.
The distance travelled by a wave in time period $T\;\text{sec} = λ$
The distance travelled by a wave in $1\;\text{sec} = \frac{λ}{T}$ \[v=\frac{λ}{T}\] \[v=fλ\] The value of $v$ depends on the elastic and inertial properties of the medium i.e. it is constant for a given medium but changes according to the nature of the medium.
Particle Speed
During the propagation of mechanical wave, the medium particles undergo up and down motions around their mean positions. The speed of these medium particles is known as particle speed. The displacement of medium particle in time $t$ is given by \[y=A\sin(ωt-kx)\] Differentiating with respect to time $t$, \[\frac{dy}{dt}=A\cos(ωt-kx)\] \[v=A\cos(ωt-kx)\] This gives the particle speed of the medium. For maximum speed, $\cos(ωt-kx)=1$, \[v_{\text{max}}=ωA\] Similarly, acceleration of the medium particle is given by \[a=\frac{d^2y}{dt^2}=-ω^2A\sin(ωt-kx)=-ω^2y\]
Amplitude
The maximum distance through which a medium particle moves either side of the mean position is called amplitude of the wave. The amplitude at the crest and the trough are identical.
Wave Number (k)
The number of waves per unit distance is called wave number $(k)$. Its unit is $\text{m}^{-1}$. Mathematically, \[\text{Wave Number}\;(k)=\frac{2π}{λ}\]
Phase of a Wave
The argument of the sine/cosine in a given wave equation is called phase. The equation of the progressive wave is, \[y=a\sin(ωt-kx)\] In this equation, $(ωt-kx)$ is phase denoted by $Φ$. \[Φ=ωt-kx\] The phase changes with both time $(t)$ and space coordinate $(x)$. When one wave is ahead of another by some angle then the difference in angle is called phase difference.
Relation between Phase Difference and Path Difference
Let us consider a complete wave of wavelength $λ$ which starts from $A$ and reaches $B$. If another wave starts from $B$ then the path difference between these two waves will be $λ$ and phase difference will be $2π$ radian.
For path difference $=λ$, phase difference $= 2π$
For path difference $= 1$, phase difference $= \frac{2π}{λ}$
For path difference $= x$, phase difference $= \frac{2π}{λ}x$
Thus, \[\text{Phase Difference} \; (Φ)= \frac{2π}{λ}x\]
More on Wave And Wave Motion