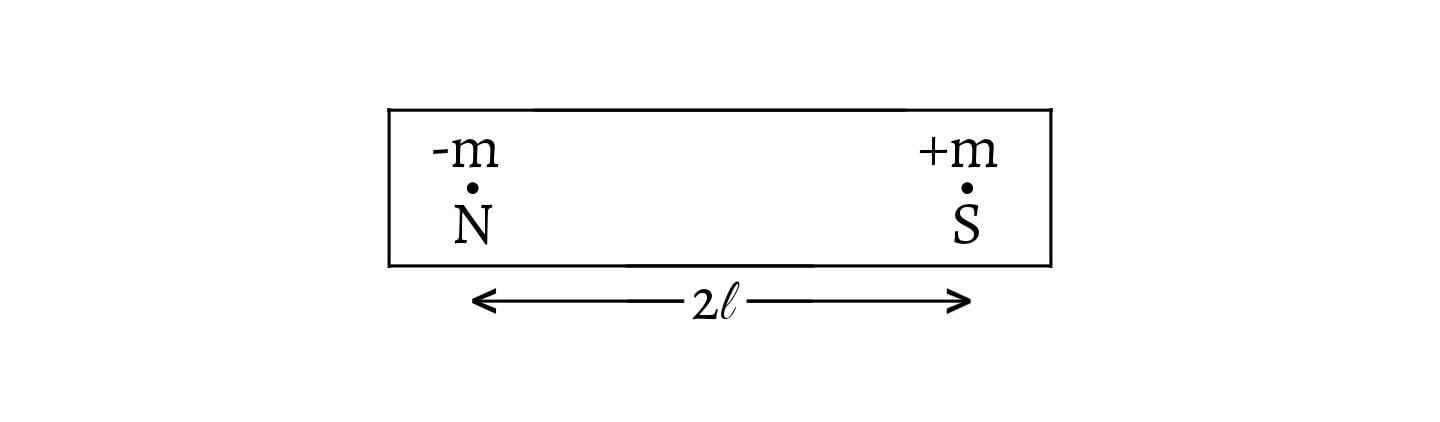Magnetic Vectors | Intensity, H-Vector and Induction Vector

There are three magnetic vectors. They are:

Intensity of Magnetization or simply Magnetization
Magnetizing Field Vector or H-vector
Magnetic Induction Vector or Magnetic Flux Density

Intensity of Magnetization or Simply Magnetization

When a magnetic material is subjected to a magnetic field, the material is magnetized. Intensity of magnetization is a measure of extent to which the material is magnetized. It depends upon the nature of the material.

Mathematically, the magnetic moment per unit volume of the material is called intensity of magnetization. It is denoted by $I$. Its direction is the same as magnetic moment.

Consider a magnetized material of magnetic moment $M$ and volume $V$. If $I$ is its intensity of magnetization, then, \[I=\frac{M}{V}\]

If the material is of length $2l$, pole strength $m$ and cross sectional area $A$, then, we can write \[I=\frac{M}{V}=\frac{m×2l}{A×2l}\] \[\therefore I=\frac{m}{A}\]

Thus, intensity of magnetization can also be defined as the pole strength per unit cross sectional area of the magnetic material. Its SI unit is Weber per metre squared $(\text{Wb m}^{-2})$ or Ampere per metre $(\text{A m}^{-1})$.

Magnetizing Field Vector or H-vector

When a magnetic substance is placed inside a magnetic field, the substance gets magnetized. The magnetic field which produces the induced magnetism in the magnetic substance placed inside it, is called magnetizing field.

The strength of magnetizing field with direction is called magnetizing field vector or magnetizing field intensity. It is denoted by $H$. It is also defined as the number of magnetic field lines due to magnetizing field passing per unit area normally through the magnetic substance.

Mathematically, \[H=ni\] Where, $n$ is the number of turns per unit length of a solenoid carrying current $i$. [Magnetic Field at a Point on the Axis of a Solenoid]

Its SI unit is Ampere per metre $(\text{Am}^{-1})$. It only depends upon current, it is independent of the medium in which the material is placed.

Magnetic Induction Vector or Magnetic Flux Density

The magnetism produced when a magnetic substance is placed inside a magnetic field is called induced magnetism and this phenomenon is called magnetic induction.

The end of the magnetic substance at which the magnetic lines of applied magnetic field enter into the substance becomes South pole while the end at which the magnetic lines leave from the substance becomes North pole. The induced magnetism also produces its own magnetic field. Hence, there are two magnetic fields inside the magnetic substance; one is the applied magnetizing field and another is the induced magnetic field.

Net Magnetic Field

The net magnetic field is given by the sum of applied magnetizing field and induced magnetic field in the material which is called magnetic induction vector or magnetic flux density. If $B$ is the net field, $B_o$ is the applied magnetizing field and $B_M$ is the induced magnetic field, then \[B=B_o+B_M\]

In the solenoid of $n$ turns per metre, \[\text{Uniform Applied Field }(B_o)=\mu_o ni\] Where, $μ_o$ is the permeability of free space and $i$ is the current in the solenoid. [Magnetic Field at a Point on the axis of a Solenoid]

Also, \[\text{Magnetizing Field Vector }(H)=ni\] \[\therefore B_o=\mu_o H\]

The magnetization field $B_M$ is related to the intensity of magnetization $I$ as \[B_M=\mu_o I\]

Hence, the net magnetic field $B$ becomes \[B=B_o+B_M\] \[B=\mu_o H+\mu_o I\] \[B=\mu_o (H+I)\]

In terms of magnetic field lines, magnetic induction vector is also defined as the number of lines passing per unit area normally through the magnetic substance due to magnetizing field as well as induced field.

Its unit in CGS system is $\text{Gauss}$ or $\text{Maxwell cm}^{-2}$ and in SI unit, its unit is $\text{Tesla}$ $(T)$, $\text{Weber metre}^{-2}$ $(\text{Wb m}^{-2})$ or Ampere per metre $(\text{Am}^{-1})$.

More on Magnetism